Number systems questions are provided here with solutions. In Class 9, we will come across the Number System chapter where we learn the basics of different types of numbers and their applications. In number theory, you may have learned about the different classifications of numbers, such as whole and natural numbers, even and odd numbers, etc. Here, we will solve the problems based on rational and irrational numbers. Students can practice the questions and it would be helpful for the students to understand this chapter. Here, we have provided a variety of number system questions and some important questions for practice. Let us learn in brief about each concept covered in this chapter before we solve a question based on it.

Also, read: Number System For Class 9

Number System Questions with Solutions

1. What are the five rational numbers between 1 and 2?

Solution: We need to find 5 rational numbers between 1 and 2

Divide and multiply both the numbers by (5+1)

Hence,

6/6 and 12/6 are rational numbers now.

Therefore, the required rational numbers between 1 and 2 are:

6/6, 7/6, 8/6, 9/6, 10/6, 11/6, 12/6.

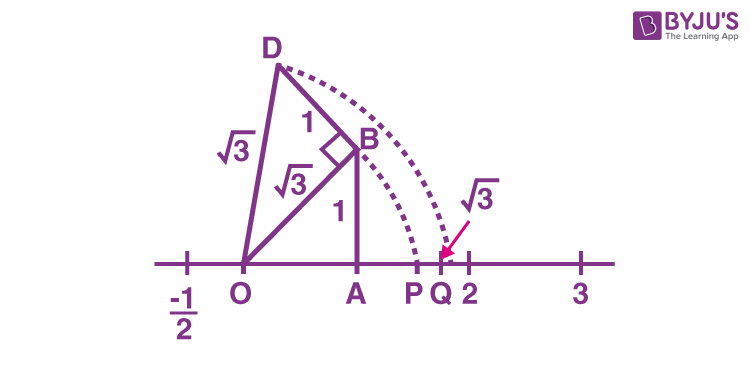
2. Can we locate √3 on the number line?

Solution: Yes, we can locate it.

Follow the steps to locate it: Construct BD of unit length perpendicular to OB

Then using the Pythagoras theorem, we see that OD = √((√2)²+1²) = √3

With centre O and radius OD, using a compass, draw an arc that cuts the number line at the point Q.

4. Show that 0.3333…, can be expressed in the form of rational number, i.e. p/q.

Solution: Let x = 0.33333

10 x = 10 × (0.333…) = 3.333…

We can write,

3.3333… = 3 + 0.3333… = 3 + x

Thus,

10 x = 3 + x

9x = 3

x=⅓

5. Write the following in decimal form and mention what expansion it is.

(i) 36/100

(ii) 1/11

Solution:

(i) 36/100 = 0.36

It is terminating.

(ii) 1/11 = 0.09090909…

It is non-terminating and repeating

6. Add 2√2 + 5√3 and √2 – 3√3.

Solution: (2√2 + 5√3) + (√2 – 3√3)

= (2+1)√2+(5-3)√3

= 3√2+2√3

7. Multiply 6√2 by 2√2.

Solution: 6√2 x 2√2

6 x 2 x √2 x √2

= 12 x 2

= 24

8. Rationalise the denominator of 1/√3

Solution: To rationalise the denominator of 1/√3, we need to multiply the numerator and denominator by √3

1/√3 x (√3/√3) = √3/3

9. Rationalise the denominator of √2/(√3-√5).

Solution: Multiply both numerator and denominator by √3+√5

[√2/(√3-√5)] x [(√3+√5)/(√3+√5)]

Numerator = √2(√3+√5)

Denominator = (√3-√5)(√3+√5) = (√3)²-(√5)² = 3-5 = -2

Therefore,

[√2(√3+√5)]/-2

= [-√2(√3+√5)]/2

10. Simplify:

(i) 2^1/3.2^2/3

(ii) (3^1/5)⁴

(iii) 7^1/3/7^1/5

(iv) 13^1/7.17^1/7

Solution:

(i) 2^1/3.2^2/3

= 2^1/3+⅔

= 2^3/3

= 2

(ii) (3^1/5)⁴

= 3^4/5

(iii) (7^1/3)/(7^1/5)

= 7^(1/3)-(1/5)

= 7^2/15

(iv) 13^1/7.17^1/7

= (13.17)^1/7

= 221^1/7

Video Lesson