Sphere
A sphere is a three-dimensional object that is round in shape. The sphere is defined in three axes, i.e., x-axis, y-axis and z-axis. This is the main difference between circle and sphere. A sphere does not have any edges or vertices, like other 3D shapes.
The points on the surface of the sphere are equidistant from the center. Hence, the distance between the center and the surface of the sphere are equal at any point. This distance is called the radius of the sphere. Examples of spheres are a ball, a globe, the planets, etc.
| Table of contents: |
What is a Sphere?
As discussed in the introduction, the sphere is a geometrical figure that is round in shape. The sphere is defined in a three-dimensional space. The sphere is three dimensional solid, that has surface area and volume. Just like a circle, each point of the sphere is at an equal distance from the center.
| Radius | The distance between surface and center of the sphere is called its radius |
| Diameter | The distance from one point to another point on the surface of the sphere, passing through the center, is called its diameter. |
| Surface area | The region occupied by the surface of the sphere is called it’s surface area |
| Volume | The amount of space occupied by any spherical object is called its volume |
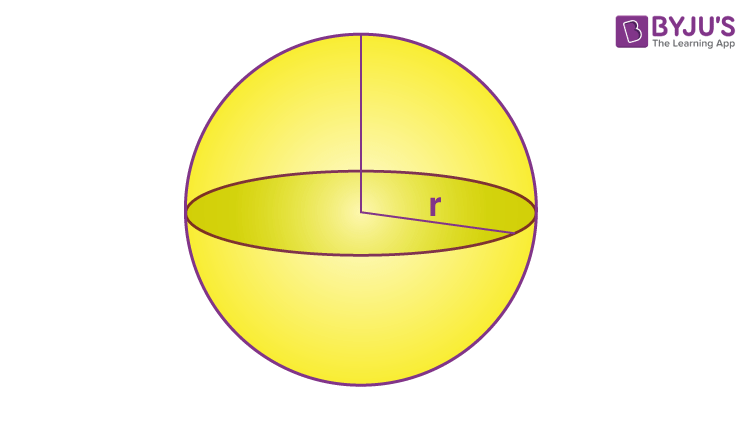
In the above figure, we can see, a sphere with radius ‘r’.
Unlike a circle, which is a plane shape or flat shape, defined in XY plane, a sphere is defined in three dimensions, i.e. x-axis, y-axis and z-axis.
Important Facts on Sphere
|
Shape of Sphere
The shape of a sphere is round and it does not have any faces. The sphere is a geometrical three-dimensional solid having a curved surface. Like other solids, such as cube, cuboid, cone and cylinder, a sphere does not have any flat surface or a vertex or an edge.
The real-life examples of the sphere are:
- Basketballs
- World Globe
- Marbles
- Planets
- Moon
Properties of a sphere
The important properties of the sphere are given below. These properties are also called attributes of the sphere.
- A sphere is perfectly symmetrical
- A sphere is not a polyhedron
- All the points on the surface are equidistant from the center
- A sphere does not have a surface of centers
- A sphere has constant mean curvature
- A sphere has a constant width and circumference.
Equation of a Sphere
In analytical geometry, if “r” is the radius, (x, y, z) is the locus of all points and (x0, y0, z0) is the center of a sphere, then the equation of a sphere is given by:
|
(x -x0)2 + (y – y0)2 + (z-z0)2 = r2 |
Sphere Formulas
The common formulas of the sphere are:
- Surface area
- Volume
| Diameter of sphere | D = 2r, where r is the radius |
| Surface area of sphere | SA = 4πr2 Square units |
| Volume of sphere | V = 4/3 πr3 Cubic Units |
Surface Area of a Sphere
The surface area of a sphere is the total area covered by the surface of a sphere in a three-dimensional space. The formula of surface are is given by:
|
The Surface Area of a Sphere(SA) = 4πr2 Square units |
Where “r” is the radius of the sphere.
Volume of a Sphere
The amount of space occupied by the object three-dimensional object called a sphere is known as the volume of the sphere.
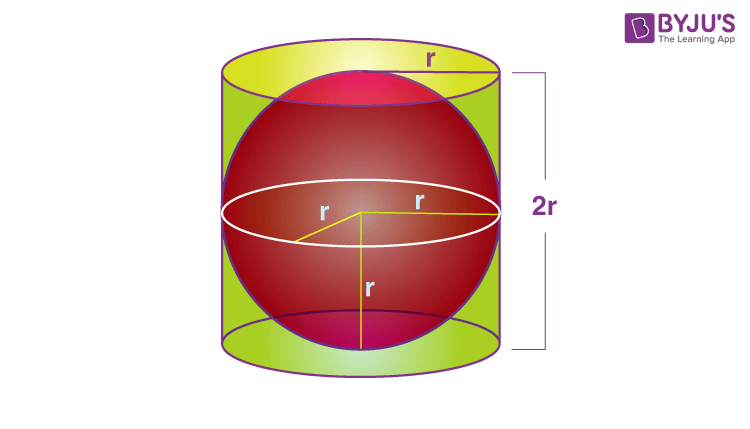
According to the Archimedes Principle, the volume of a sphere is given as,
|
The volume of Sphere(V) = 4/3 πr3 Cubic Units |
Difference Between a Sphere and a Circle
A circle and a sphere are shapes in geometry, that appear the same, but are different in properties. The key differences between the two shapes are listed below in the table.
| Circle | Sphere |
| A circle is a two-dimensional or 2d shape | A sphere is a three-dimensional or 3d shape |
| A circle is defined by two axes, the x-axis and the y-axis. | A sphere is defined by three axes, x-axis, y-axis and z-axis |
| The region occupied by a circle is simply an area.
The formula of the area is πr2 |
A sphere has a surface area covered by its outer surface, which is equal to 4πr2 |
| It does not have any volume | It has volume |
| It has a flat face | It has no flat face but a curved face |
Related Articles on Sphere
- Area & Volume Of Sphere
- Difference between Circle and Sphere
- Equation of Sphere
- Surface Area of a Hemisphere
- Volume of Hemisphere
Solved Examples on Sphere
Example 1: Find the volume of the sphere that has a diameter of 10 cm?
Solution:
Given, Diameter, d = 10 cm
We know that D = 2 r units
Therefore, the radius of a sphere, r = d / 2 = 10 / 2 = 5 cm
To find the volume:
The volume of sphere = 4/3 πr3 Cubic Units
V = (4/3)× (22/7) ×53
Therefore, the volume of sphere, V = 522 cubic units
Example 2: Determine the surface area of a sphere having a radius of 7 cm.
Solution:
Given radius = 7 cm
The Surface Area of a Sphere(SA) = 4πr2 Square units
SA = 4× (22/7)× 72
SA = 4 × 22 × 7
SA = 616 cm2
Therefore, the surface area of a sphere = 616 square units.
Practice Questions
- Find the volume of the sphere if diameter = 10cm.
- If the radius of a sphere is 14 cm, then find its surface area.
- A cricket ball with radius ‘r’ cm and a basketball with radius ‘4r’ have volume in the ratio of?
- Metallic spheres of radii 3 cm, 4 cm and 5 cm, respectively, are melted to form a single solid sphere. Find the radius of the resulting sphere.
Register with BYJU’S – The Learning App to learn about other three-dimensional shapes also watch interactive videos to learn with ease.
Frequently Asked Questions on Sphere
What is a sphere?
How many sides does a sphere have?
Is a sphere, circle?
What is Hemisphere?
What are the characteristics of a sphere?
What are the examples of spheres?
What is surface area and volume of sphere?
SA = 4πr2 Square units
Volume of sphere is the space occupied by sphere in three dimension space. The formula is:
V = 4/3πr3