Volume of cuboid is the total space occupied by the cuboid in a three-dimensional space. A cuboid is a three-dimensional structure having six rectangular faces. These six faces of the cuboid exist as a pair of three parallel faces. Therefore, the volume is a measure based on the dimensions of these faces, i.e. length, width and height. It is measured in cubic units. Surface area of cuboid is the total area covered by its rectangular faces.

In this article, let us discuss what is a volume of a cuboid, its formula, along with the volume of a cuboid prism and a cube example.

What is the Volume of a Cuboid?

The volume of a Cuboid, in general, is equal to the amount of space occupied by the shape of cuboid.  It depends on the three dimensions of cuboid, i.e., length, breadth and height. The term “Solid Rectangle” is also known as a cuboid, because all the faces of a cuboid are rectangular.  In a rectangular cuboid, all the angles are at right angles and the opposite faces of a cuboid are equal.

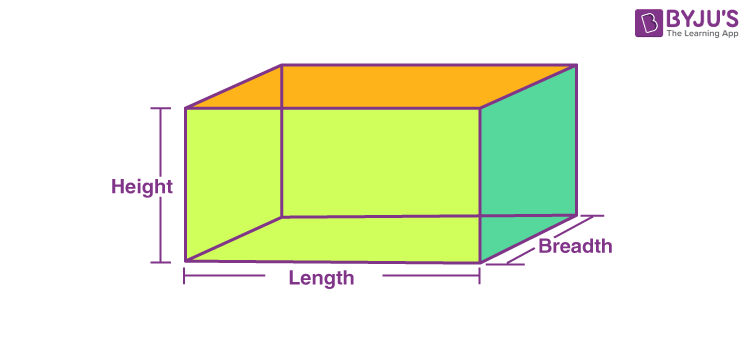
Volume of Cuboid Formula

The volume of a cuboid is given by the product of its dimensions, i.e., length, width and height. The unit of volume of cuboids is cubic units or unit³, such as m³, cm³, in³, etc.

Volume of cuboid is equal to product of its base area and height. Hence, we can write;

Volume of cuboid = Base area × Height  [Cubic units]

The base of the cuboid is rectangle in shape. So, the base area of a cuboid is equal to the product of its length and breadth. Hence,

Volume of a cuboid = length × breadth × height    [cubic units]

or

Volume of a cuboid = l × b × h    [cubic units]

Where,

• l = length
• b = breadth
• h = height

Also, try: Volume of Cuboid Calculator

How to Find Volume of Cuboid?

Volume of a cuboid is the space occupied by its dimensions, inside the cuboid. These dimensions are length, width and height. When the area of the faces of a cuboid is the same, we call this cuboid a cube. The area of all the faces of a cube is the same as they are all squares.

Think of a scenario where we need to calculate the amount of sugar that can be accommodated in a cuboidal box. In other words, we mean to calculate the volume of this box. The capacity of a cuboidal box is basically equal to the volume of the cuboid. Thus, if we know, the length, width and height of the cuboidal box, we can easily measure volume using the given formula:

Volume of cuboidal box = Length x Width x Height

Follow the below steps to find the volume of any cuboidal shape:

• Step 1: Check the dimensions of the given cuboid, i.e., length, width and height
• Step 2: Check if all the dimensions are of the same units, else we need to convert them into the same units
• Step 3: After we have made units the same for all the dimensions, multiply length, width and height together.
• Step 4: The obtained value is the volume of cuboids, written with cubic units.

Video Lesson on Volume of Cuboid

For More Information On Volumes of Cubes and Cuboid, Watch The Below Video:

Total Surface Area of Cuboid

The total surface area of a cuboid is equal to the sum of the areas of the six rectangular faces whereas the Lateral surface area of a cuboid equal to the sum of the four rectangular faces, in which two rectangular faces of the top and bottom faces are excluded. The formula for the total surface area and lateral surface area of a cuboid is given as:

Total Surface Area of a Cuboid = 2 (lb + hb + lh) square units

Lateral Surface Area of a Cuboid = 2h (l+b)

Now, let us discuss the volume of a cuboid in detail.

Volume of a Cuboid Prism

A cuboid prism or a rectangular prism is the same as a cuboid. It has 6 faces, 8 vertices, and 12 edges. When a cuboid prism or a rectangular prism has a rectangular cross-section. A prism is called a right prism when the angle between the base and the sides are at right angles. Also, the top and the bottom surfaces are in the same shape and size.  The volume of the cuboid prism is given as:

Volume of a cuboid prism or rectangular prism, V= length × breadth × height (cubic units)

Volume of a Cube

Volume of cube: Cuboid in which length of each edge is equal is known as a cube. Thus,

Volume of a cube of side ‘a’ = a³

Solved Examples on Volume of Cuboid

Question 1: Find the volume of a cuboid whose length = 5 cm, width = 2 cm and height = 3 cm.

Solution: Given,

length = 5 cm, width = 2 cm and height = 3 cm

By the formula, we know;

Volume of cuboid = length x width x height

= 5 cm x 2 cm x 3 cm

= 30 cu.cm.

Question 2: Calculate the amount of air that can be accumulated in a room that has a length of 5 m, breadth of 6 m and a height of 10 m.

Solution: Amount of air that can be accumulated in a room = capacity of the room = volume of a cuboid

Volume of cuboid = l × b × h = 5 ×6 ×10 = 300 m³

Thus, this room can accommodate the maximum of 300 m³ of air.

Practice Questions

Find the volume of cuboid with following dimensions:

1. Length = 15 cm, Breadth = 50 cm and Height = 22 cm
2. Length = 7 m, Breadth = 3 m and Height = 5 m
3. Length = 2 m, Breadth = 2.5 m and Height = 1.5 m
4. Length = 80 cm, Breadth = 20 cm and Height = 44 cm
5. Length = 1.7 m, Breadth = 1.5 m and Height = 1 m

To learn and practice more problems in the surface area of cuboid, you can visit BYJU’S – The Learning App