A pyramid is defined as a three-dimensional structure encompassing a polygon as its base. You must have heard about The Great Pyramid of Giza, which is structured in the same concept. Every corner of this structure is linked to a single apex which makes it appear as a distinct shape. In this article, we are going to discuss what is a pyramid shape, different types of the pyramid with formulas and many solved examples.

Table of Contents:

• Pyramid Shape
• Types of Pyramid
• Right Vs Oblique Pyramid
• Regular Vs Irregular Pyramid
• Pyramid Formula
• Examples
• FAQs

Pyramid Shape

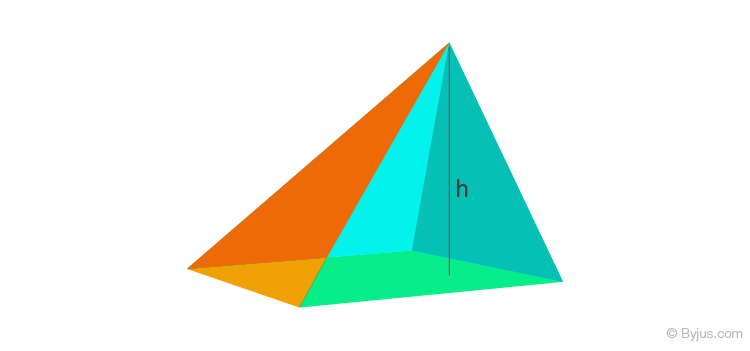
A pyramid is a three-dimensional shape. A pyramid has a polygonal base and flat triangular faces, which join at a common point called the apex. A pyramid is formed by connecting the bases to an apex. Each edge of the base is connected to the apex, and forms the triangular face, called the lateral face. If a pyramid has an n-sided base, then it has n+1 faces, n+1 vertices, and 2n edges.

Types Of Pyramids

Based on the shape of the base, the pyramid is classified into different types. Now, let us discuss the different types of pyramid shape one by one.

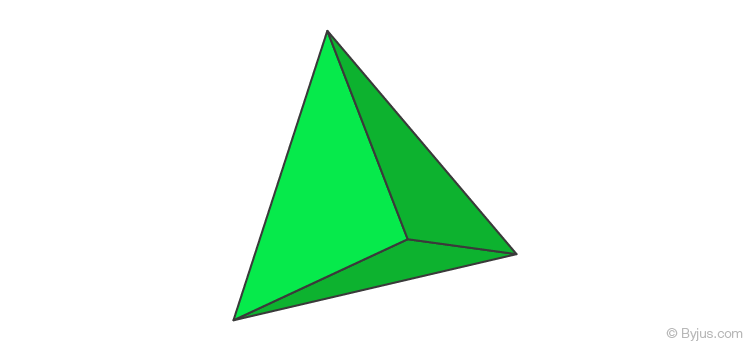
Triangular Pyramid

If the base of the pyramid is in a triangular shape (base with 3 sides), then the pyramid is called a triangular pyramid. As the triangle has 3 sides, then the triangular pyramid has the following properties:

No. of Faces = (3+1) = 4

No.of Vertices: (3+1) = 4

No. of Edges: 2(3) = 6

Square Pyramid

If the base of the pyramid is in the shape of a square (base with 4 sides), then it is called a square pyramid. As the square has 4 sides, then the square pyramid has the following properties:

No. of Faces: (4+1) = 5

No.of Vertices: (4+1)= 5

No. of Edges: 2(4) = 8

Pentagonal Pyramid

If the base of the pyramid is in the shape of a pentagon (base with 5 sides), then it is called a pentagonal pyramid. As the pentagon has 5 sides, then the pentagonal pyramid has the following properties:

No. of Faces: (5+1)=6

No.of Vertices: (5+1)=6

No. of Edges: 2(5)= 10

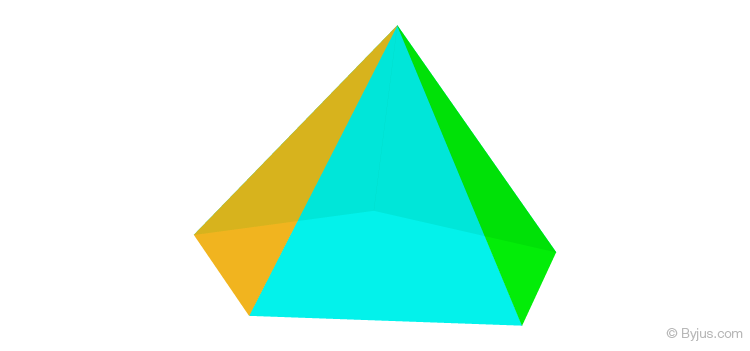
Right Pyramid Vs Oblique Pyramid

Right Pyramid:

The apex of this pyramid is exactly over the middle of the base, hence named as Right Pyramid.

Oblique Pyramid

The apex of this pyramid is not exactly over the middle of its base and named as Oblique Pyramid.

Regular vs Irregular Pyramid

To distinguish between regular and irregular Pyramid, you need to consider the shape of the base. If the base of a polygon is regular, it is labelled as Regular Pyramid, else it is considered as Irregular Pyramid. The figure given below illustrates the regular pyramid and an irregular pyramid.

           

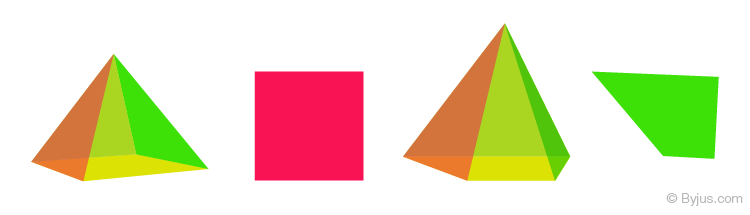
                           Regular                                                                                             Irregular

Pyramid Formulas

The standard formula to find the surface area and the volume of the pyramid are given as follows:

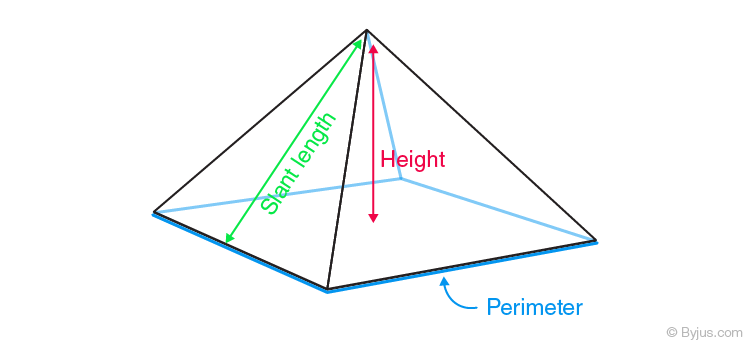
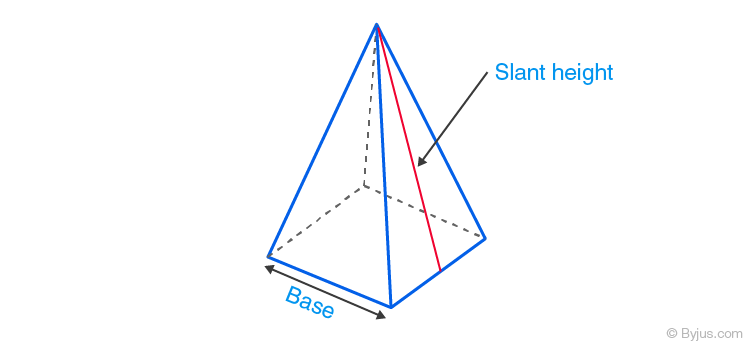
The total surface area of a pyramid is the sum of the base area and half the product of the base perimeter and the slant height.

Thus,

The Total Surface Area of Pyramid = (½)Pl +B square units

Where, 

“P” is the perimeter of the base

“l” is the slant height 

“B” is the base area.

The general form to find the volume of the pyramid is one-third of the base area and the height of the pyramid.

Thus,

The volume of the pyramid = (⅓)×(Base Area)×(Height) Cubic units.

Solved Examples on Pyramid

Example 1: 

Find the volume of the square pyramid, if its base area is 56 cm² and its height is 9 cm.

Solution:

Given: 

The base area of the square pyramid = 56 cm²

Height = 9 cm

Thus, the volume of the square pyramid = (⅓)(Base area)(Height) cubic units

Now, substitute the values in the formula, we get

The volume of a square pyramid = (⅓)(56)(9)

V = (56)(3)

V= 168 cm³

Hence, the volume of the square pyramid is 168 cm³.

Example 2:

Find the total surface area of the square pyramid if each side of the base measures 16 cm, and the slant height is 17 cm, and the altitude is 15 cm.

Solution:

Given:

As the base is a square, the perimeter of the base is 4 times 16 cm

P =  4(16)

P = 64 cm

The area of the base = a²

B = 16² = 256 cm²

We know, that the total surface area of the square pyramid is (½)Pl +B square units

Substituting the values in the given formula, we get

The total surface area of the square pyramid = [(½)(64)(17)] + (256)

TSA = 544 + 256

TSA = 800 cm²

Hence, the total surface area of the square pyramid is 800 cm².

Keep visiting us and download BYJU’S – The Learning App to continue learning more maths concepts in an engaging and effective way.