In Maths, reciprocal is simply defined as the inverse of a value or a number. If n is a real number, then its reciprocal will be 1/n. Thus, here we convert the number into an upside-down form. For example, the reciprocal of 9 is 1 divided by 9, i.e. 1/9. Now, if we multiply a number by its reciprocal, it gives a value equal to 1. Thus, it is also called multiplicative inverse. The word reciprocal came from the Latin word “reciprocus” meaning “returning”. Hence, it returns its original value, if we take the reciprocal of an inverted number. In this article, we are going to learn the definition of reciprocal, how to find the reciprocal of numbers, fractions and decimals with many examples.

Definition

In Mathematics, reciprocal means an expression which when multiplied by another expression, gives unity (1) as a result. The reciprocal of any quantity is, one divided by that quantity. For any number ‘a’, the reciprocal will be 1/a.

Example: Reciprocal of a number 7 is 1/7.

Therefore, a reciprocal is a flipped number that can bring back the original number.

Other Definitions of Reciprocal

It has many other definitions too :

• It is also called the multiplicative inverse.
• It is similar to turning the number upside down.
• It is also found by interchanging the numerator and denominator.
• All the numbers have reciprocal except 0.
• The product of a number and its reciprocal is equal to 1.
• Generally, reciprocal is written as, 1/x or x^-1 for a number x.

Example

Examples: The reciprocals of 3 and  8 are 1/3 and 1 /8

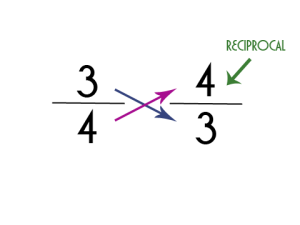
The reciprocal of 3/4 is 4/3.

It is also expressed by the number raised to the power of negative one and can be found for fractions and decimal numbers too.

In maths, when you take the reciprocal twice, you will get the same number that you started with.

Example:  The reciprocal of 4 is 1/4. When you repeat this step it becomes 4/1 or 4. Here you get the same number where you started with.

Not For Zero

We cannot apply the reciprocal condition on zero, since it will return an indefinite value. 

1/0 = Undefined

Therefore, we can have reciprocal for all real numbers but not for zero.

Reciprocal of a Number

It is defined as one over that number.

Example: Find the reciprocal of 5

Solution: To find the solution, we will use x=1/x

Therefore, 5= 1/5

The reciprocal of a function, f(x) = f(1/x)

Reciprocal of Negative Numbers

For any negative number -x, the reciprocal can be found by writing the inverse of the given number with a minus sign along with that (i.e) -1/x. For example, the reciprocal of – 4x² is written as -1/4x². Go through the following steps to find the reciprocal of the negative number.

Step 1: For any negative number, write the given number in the form of an improper fraction by writing the number 1 in the denominator.

Step 2: Now, interchange the numerator and denominator values.

Step 3: Add a minus sign (-) to the resultant number.

Now, Consider a negative number, -17.

Step 1: Convert the number 17 in the improper fraction. (i.e) 17/1.

Step 2: Interchanging the numerator and denominator value, we get 1/17.

Step 3: Finally, adding a negative sign to the resultant number, we get -1/17.

Therefore, the reciprocal of -17 is -1/17.

Reciprocal of a Fraction

The reciprocal of a fraction can be found by interchanging the numerator and the denominator values.

Example: Find the reciprocal of 2 / 3

Solution: To find the solution we will follow the following steps

The reciprocal of 2/3 is 3/2. (or) use the formula, x = 1/x, where 2/3=1/2/1/3

Therefore, the reciprocal of a fraction 2/3 is 3/2.

Reciprocal of a Mixed Fraction

In order to find the same for a mixed fraction, convert it into improper fractions and do the operation.

Consider a mixed fraction, 4(1/2).

The first step is to convert a mixed fraction into an improper fraction.

4(1/2)= 1+1+1+1+1/2

= (2/2) + (2/2) + (2/2) + (2/2) + (1/2)

= (2+2+2+2+1)/2

=9/2

4(1/2) = 9/2

Now, you get the fraction and do the same operation for finding the reciprocal by flipping the numerator and the denominator.

Therefore, the solution for 9/2 is 2/9.

Reciprocal of a Decimal

The reciprocal of a decimal is the same as it is for a number defined by one over the number.

Example: Find the reciprocal of a decimal 0.75

Solution: The reciprocal of a number, x=1/x

Therefore, 0.75= 1/0.75

An alternate method to find it is given below.

Consider the same example, 0.75.

First, you have to check whether the given decimal number is possible for converting into a fractional number. Here 0.75 is written as 3/4

Now, find the reciprocal of 3/4 which gives 4/3

When you verify both the solutions, it results in the same.

That is, 1/0.75 = 1.33 and

4/3 = 1.33

Finding Unity

If we multiply the reciprocal of a number by the number itself, we will get the value equal to unity (1). Let us see some examples here:

• 2 x ½ = 1
• 3 x ⅓ = 1
• 10 x 1/10 = 1
• 50 x 1/50 = 1
• 100 x 1/100 = 1

From the above examples, we can see that the multiplication of a number to its reciprocal gives 1. Therefore, it defines reciprocal as a value to be multiplied by another value to get 1.

Solved Examples

Go through the below examples:

Example 1:

Find the reciprocal of 2 and 9

Solution:

Given that, the two integers are 2 and 9

Therefore, the reciprocal of 2 is ½

The reciprocal of 9 is 1/9

Example 2:

Determine the reciprocal of 3/(2/3)

Solution:

Given number 3/(⅔) is a fraction.

Therefore, the reciprocal of 3/(⅔) is 3 × (3/2)

 3/(⅔) = 9/2

Hence, the reciprocal of  9/2 is 2/9.

Example 3:

Write down the opposite reciprocal of 5/4.

Solution:

Given fraction is 5/4

The reciprocal of 5/4 is 4/5.

Hence, the opposite reciprocal of 5/4 is – 4/5.

Example 4: 

Find the reciprocal of the decimal 0.25. 

Solution:

Given decimal number = 0.25.

Hence, the reciprocal of 0.25 is 1/0.25.

Alternate method:

The fraction equivalent to the decimal number 0.25 is 1/4.

Hence, the reciprocal of ¼ is 4/1.

Verification:

The resultant number obtained from both methods will result in the same value.

(i.e.) 1/0.24 = 4 or 4/1.

Example 5: 

Find the reciprocal of the negative number -45.

Solution:

Given that the negative number is -45.

Hence, the reciprocal of -45 is -1/45.

Reciprocal – Practice Questions

Find the reciprocal for the following numbers:

1. 29
2. 14/15
3. 1.25
4. -80
5. ax²

For more information on finding reciprocals and other maths concepts, visit BYJU’S and also get various maths related videos to understand the concept in an easy and engaging way.