Before knowing about a sector of a circle, let’s know how the area of a circle is calculated. When it comes to the area, it is always related to two-dimensions. Anything which is two dimensional can form a plane. So, any two-dimensional figure will have area. What about a circle?

Definition 1: A circle is the collection of all the points in a plane which are at a fixed distance from a fixed point. The fixed point is known as the center of the circle and the fixed distance is known as the radius of the circle.

Definition 2: If all the points which lie inside and on the circle are taken together, the plane constructed is known as a disk.

A disk is basically the region bounded by a circle. So, the area of a circle will always be that of the disk. The area, A of the circle with radius r is given by

\(A\) = \(π~r^2\)

Definition 3: The portion of the circle enclosed by two radii and the corresponding arc is known as the sector of a circle.

Basically, a sector is the portion of a circle. It would hence be right to say that a semi-circle or a quarter-circle is a sector of the given circle. In fig.1, OPAQ is called the minor sector and OPBQ is called the major sector because of lesser and greater areas. The angles subtended by the arcs PAQ and PBQ are equal to the angle of the sectors OPAQ and OPBQ respectively. When the angle of the sector is equal to 180°, there is no minor or major sector.

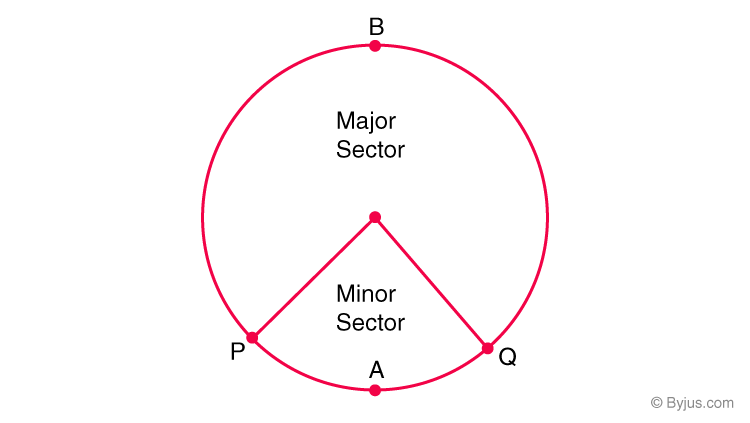
Area of sector

In a circle with radius r and center at O, let ∠POQ = θ (in degrees) be the angle of the sector. Then, the area of a sector of circle formula is calculated using the unitary method.

 

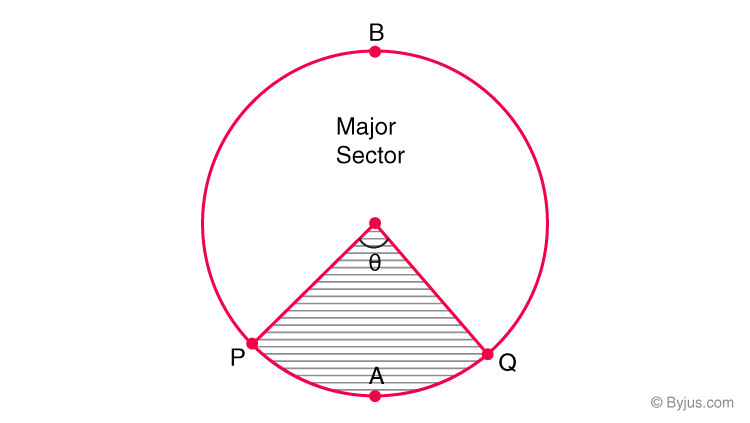
When angle of the sector is 360°, area of the sector i.e. the whole circle = \(πr^2\)

When the angle is 1°, area of sector = \(\frac{πr^2}{360°}\)

So, when the angle is θ, area of sector, OPAQ,

\(A\) = \(\frac{θ}{360°}~×~ πr^2\)

Similarly, length of the arc (PQ) of the sector with angle θ,

l = \(\frac{θ}{360°}×2πr\)

If the length of the arc of the sector is given instead of the angle of the sector, there is a different way to calculate the area of the sector. Let the length of the arc be l. For the radius of a circle equal to r units, an arc of length r units will subtend 1 radian at the center. It can be hence concluded that an arc of length l will subtend \(\frac{l}{r}\) angle at the center. So, if l is the length of the arc, r is the radius of circle and θ is the angle subtended at center,

\(θ\) = \(\frac{l}{r}\), where θ is in radians

When angle of the sector is 2π, area of the sector i.e. the whole circle = \(πr^2\)

When the angle is 1, area of the sector = \(\frac{πr^2}{2π}\) = \(\frac{r^2}{2}\)

So, when the angle is θ, area of the sector = \(θ~×~\frac{r^2}{2}\)

= \(\frac{l}{r}~×~\frac{r^2}{2}\)

= \(\frac{lr}{2}\)

Some examples for better understanding are discussed from here on.

Example 1: If the angle of the sector with radius 4 units is 45°, area = \(\frac{θ}{360°}~×~ πr^2\)

= \(\frac{45°}{360°}~×~\frac{22}{7}~×~4~×~4\)

= \(\frac{44}{7}\) square units

The length of the same sector = \(\frac{θ}{360°}~×~ 2πr\)

= \(\frac{45°}{360°}~×~2~×~\frac{22}{7}~×~4\)

= \(\frac{22}{7}\) units

Example 2: If the length of the arc of a circle with radius 16 units is 5 units, the area of the sector corresponding to that arc = \(\frac{lr}{2}\) = \(\frac{5~×~16}{2}\) = \(40\) square units

To practice more on are of sector of a circle, download BYJU’S – The Learning App from the Google Play Store.