Complement of a Set
Before studying about the Complement of a set, let us understand what are sets?
Sets Definition
A well-defined collection of objects or elements is known as a set. Any set consisting of all the objects or elements related to a particular context is defined as a universal set. It is represented by \(U\).
For any set A which is a subset of the universal set \(U\), the complement of the set \(A\) consists of those elements which are the members or elements of the universal set \(U\) but not of the set \(A\). The complement of any set \(A\) is denoted by \(A'\).
Also, read:
Complement of a Set Definition
If \(U\) is a universal set and \(A\) be any subset of \(U\) then the complement of \(A\) is the set of all members of the universal set \(U\) which are not the elements of \( A\).
\(A'\) = {\({x ~:~ x~ ∈ ~U ~and ~x ~∉ ~A}\)}
Alternatively it can be said that the difference of the universal set \(U\) and the subset \(A\) gives us the complement of set \(A\).
Venn Diagram for the Complement of a set
The Venn diagram to represent the complement of a set A is given by:
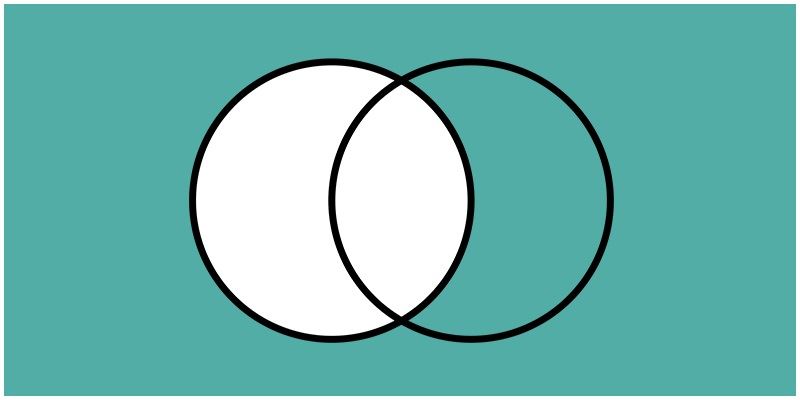
Complement of a Set Examples
To make it more clear consider a universal set \(U\) of all natural numbers less than or equal to 20.
Let the set \(A\) which is a subset of \(U\) be defined as the set which consists of all the prime numbers.
Thus we can see that \(A\) = {\({2, 3, 5, 7, 11, 13, 17, 19}\)}
Now the complement of this set A consists of all those elements which is present in the universal set but not in \(A\). Therefore, \(A'\) is given by:
\(A'\)={\({1,4,6,8,9,10,12,14,15,16,18,20}\)}
Example: Let \(U\) be the universal set which consists of all the integers greater than 5 but less than or equal to 25. Let \(A\) and \(B\) be the subsets of \(U\) defined as:
\(A\) = {\({x~:x~ ∈~U ~and~ x~ is~ a~ perfect~ square}\)}
\(B\) = \({7, 9, 16, 18, 24}\)
Find the complement of sets A and B and the intersection of both the complemented sets.
Solution: The universal set is defined as:
\(U\) = {\({6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25}\)}
Also, \(A\) = {\({9,16,25}\)} and
\(B\) = {\({7,9,16,18,24}\)}
The complement of set A is defined as:
\(A'\) = {\({x~:~x~∈~U~ and ~x~∉~A}\)}
Therefore, \(A'\) = {\({6,7,8,10,11,12,13,14,15,17,18,19,20,21,22,23,24}\)}
Similarly the complement of set B can be given by:
\(B'\) = {\({6,8,10,11,12,13,14,15,17,19,20,21,22,23,25}\)}
The intersection of both the complemented sets is given by \(A’∩ B'\).
Rightarrow A’∩ B’= {6, 8, 10, 11, 12, 13, 14, 15, 17, 19, 20, 21, 22, 23}
We can see from the above discussions that if a set \(A\) is a subset of the universal set \(U\) then the complement of set \(A\) i.e. \(A'\) is also a subset of \(U\)..
Now we are clear on the concept of the complement of sets. There is a lot more to learn. Enrich your knowledge and reach new horizons of success by downloading BYJU’S – The Learning App to know more or visit our website.