Partial Fractions
Partial fractions are the fractions used for the decomposition of a rational expression. When an algebraic expression is split into a sum of two or more rational expressions, then each part is called a partial fraction. Hence, basically, it is the reverse of the addition of rational expressions. Similar to fractions, a partial fraction will have a numerator and denominator, where the denominator represents the decomposed part of a rational function.
In mathematics, we can see many complex rational expressions. If we try to solve the problems in a complex form, it will take a lot of time to find the solution. To avoid this complexity, we have to continue the problem by reducing the complex form of the rational expression into the simpler form. Partial fraction decomposition is one of the methods, which is used to decompose rational expressions into simpler partial fractions. This process is more useful in the integration process. In this article, you will learn the definition of the partial fraction, partial fraction decomposition, partial fractions of an improper fraction with solved examples in detail.
| Table of Contents: |
What is a Partial Fraction?
An algebraic fraction can be broken down into simpler parts known as “partial fractions“. Consider an algebraic fraction, (3x+5)/(2x2-5x-3). This expression can be split into simple form like (2)/(x-3) – (1)/(2x+1).
The simpler parts [(2)/(x-3)]-[(1)/(2x+1)] are known as partial fractions.
This means that the algebraic expression can be written in the form as given in the figure:
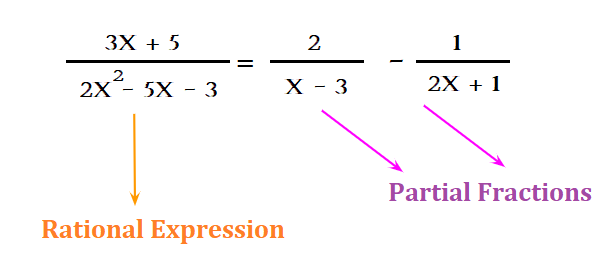
Note: The partial fraction decomposition only works for the proper rational expression (the degree of the numerator is less than the degree of the denominator). In case, if the rational expression is in an improper rational expression (the degree of the numerator is greater than the degree of the denominator), first do the division operation to convert into proper rational expression. This can be achieved with the help of a polynomial long division method.
Partial Fraction Formulas
Here the list of Partial fractions formulas is given. These formulas will help us to decompose a rational expression into partial fractions. These are common types of partial fractions which are used to solve problems.
| S.No | Rational Fraction | Partial Fraction Form |
| 1 | \(\large \frac{p(x) + q}{(x-a)(x-b)}\) | \(\large \frac{A}{x-a} + \frac{B}{(x-b)}\) |
| 2 | \(\large \frac{p(x) + q}{(x-a)^{2}}\) | \(\large \frac{A_{1}}{x-a} + \frac{A_{2}}{(x-a)^{2}}\) |
| 3 | \(\large \frac{px^{2} + qx +r}{(x-a)(x-b)(x-c)}\) | \(\large \frac{A}{x-a} + \frac{B}{(x-b)} + \frac{C}{(x-c)}\) |
| 4 | \(\large \frac{px^{2} + q(x) +r}{(x-a)^{2}(x-b)} \) | \(\large \frac{A_{1}}{x-a} + \frac{A_{2}}{(x-a)^{2}} + \frac{B}{(x-b)}\) |
| 5 | \(\large \frac{px^{2} + qx +r}{(x-a)(x^{2}+bx+c)}\) | \(\large \frac{A}{x-a} + \frac{Bx+C}{x^{2}+bx+c}\) |
Here A, B and C are real numbers.
Partial Fractions of Rational Functions
Any number which can be easily represented in the form of p/q, such that p and q are integers and q≠0 is known as a rational number. Similarly, we can define a rational function as the ratio of two polynomial functions P(x) and Q(x), where P and Q are polynomials in x and Q(x)≠0. A rational function is known as proper if the degree of P(x) is less than the degree of Q(x); otherwise, it is known as an improper rational function. With the help of the long division process, we can reduce improper rational functions to proper rational functions. Therefore, if P(x)/Q(x) is improper, then it can be expressed as:
\(\frac{P(x)}{Q(x)}= A(x) + \frac{R(x)}{Q(x)}\)
Here, A(x) is a polynomial in x and R(x)/Q(x) is a proper rational function.
We know that the integration of a function f(x) is given by F(x) and it is represented by:
∫f(x)dx = F(x) + C
Here R.H.S. of the equation means integral of f(x) with respect to x and C is the constant of integration.
Decomposition of Partial Fractions
In order to integrate a rational function, it is reduced to a proper rational function. The method in which the integrand is expressed as the sum of simpler rational functions is known as decomposition into partial fractions. After splitting the integrand into partial fractions, it is integrated accordingly with the help of traditional integrating techniques.
The procedure for finding the partial fraction decomposition is explained with steps here::
- Step 1: While decomposing the rational expression into the partial fraction, begin with the proper rational expression.
- Step 2: Now, factor the denominator of the rational expression into the linear factor or in the form of irreducible quadratic factors (Note: Don’t factor the denominators into the complex numbers).
- Step 3: Write down the partial fraction for each factor obtained, with the variables in the numerators, say A and B.
- Step 4: To find the variable values of A and B, multiply the whole equation by the denominator.
- Step 5: Solve for the variables by substituting zero in the factor variable.
- Step 6: Finally, substitute the values of A and B in the partial fractions.
Hence, the expression is decomposed into partial fractions.
Partial Fraction of Improper Fraction
An algebraic fraction is improper if the degree of the numerator is greater than or equal to that of the denominator. The degree is the highest power of the polynomial. Suppose, m is the degree of the denominator and n is the degree of the numerator. Then, in addition to the partial fractions arising from factors in the denominator, we must include an additional term: this additional term is a polynomial of degree n − m.
Note:
- A polynomial with zero degree is K, where K is a constant
- A polynomial of degree 1 is Px + Q
- A polynomial of degree 2 is Px2+Qx+K
Partial Fraction in Integration
Let us look into an example to have a better insight into integration using partial fractions.
Example: Integrate the function \(\frac{1}{(x-3)(x+1)}\) with respect to x.
Solution: The given integrand can be expressed in the form of partial fraction as:
\(\frac{1}{(x-3)(x+1)} = \frac{A}{(x-3)} + \frac{B}{(x+1)}\)
To determine the value of real coefficients A and B, the above equation is rewritten as:
1= A(x+1)+B(x-3)
⇒1=x(A+B)+A-3B
Equating the coefficients of x and the constant, we have
A + B = 0
A – 3B = 1
Solving these equations simultaneously, the value of A =1/4 and B = -1/4. Substituting these values in equation 1, we have
\(\frac{1}{(x-3)(x+1)} = \frac{1}{4(x-3)} + \frac{-1}{4(x+1)}\)
Integrating with respect to x we have;
\(\int \frac{1}{(x-3)(x+1)} = \int \frac{1}{4(x-3)} + \int \frac{-1}{4(x+1)}\)
According to the properties of integration, the integral of the sum of two functions is equal to the sum of integrals of the given functions, i.e.,
∫[f(x) +g(x)]dx = ∫f(x)dx + ∫g(x)dx
Therefore,
\(= \frac{1}{4} \int \frac{1}{(x-3)} – \frac{1}{4} \int \frac{1}{(x+1)}\)
\(= \frac{1}{4} \ln \left | x-3 \right | – \frac{1}{4} \ln \left | x+1 \right |\)
\(= \frac{1}{4} \ln \left | \frac{x-3}{x+1} \right |\)
Related Articles
Partial Fractions – Solved Examples
Example 1: Write the partial fraction decomposition of the following expression.
(20x + 35)/(x + 4)2
Solution:
(20x + 35)/(x + 4)2
(20x + 35)/(x + 4)2 = [A/(x + 4)] + [B/(x + 4)2]
(20x + 35)/(x + 4)2 = [A(x + 4) + B]/ (x + 4)2
Now, equating the numerators,
20x + 35 = A(x + 4) + B
20x + 35 = Ax + 4A + B
20x + 35 = Ax + (4A + B)
By equating the coefficients,
A = 20
4A + B = 35
4(20) + B = 35
B = 35 – 80 = -45
Therefore, (20x + 35)/(x + 4)2 = [20/(x + 4)] – [45/(x + 4)2]
Example 2: Decompose the given expression into partial fractions.
(x2 + 1)/ (x3 + 3x2 + 3x + 2)
Solution:
(x2 + 1)/ (x3 + 3x2 + 3x + 2)
Using the factor theorem, x + 2 is a factor of x3 + 3x2 + 3x + 2.
Thus, x3 + 3x2 + 3x + 2 = (x + 2)(x2 + x + 1)
Now, the given expression can be written as:
(x2 + 1)/ (x3 + 3x2 + 3x + 2) = (x2 + 1)/ [(x + 2)(x2 + x + 1)]
By the method of decomposition,
(x2 + 1)/(x + 2)(x2 + x + 1) = [A/(x + 2)] + [(Bx + C)/(x2 + x + 1)]
(x2 + 1)/(x + 2)(x2 + x + 1) = [A(x2 + x + 1) + (Bx + C)(x + 2)]/ [(x + 2)(x2 + x + 1)]
= [(A + B)x2 + (A + 2B + C)x + A + 2C]/ [(x + 2)(x2 + x + 1)]
Equating the coefficients in the numerators of both LHS and RHS,
A + B = 1
A + 2B + C = 0
A + 2C = 1
Solving these equations,
A = 5/3, B = -2/3 and C = -1/3
(x2 + 1)/(x + 2)(x2 + x + 1) = [5/3(x + 2)] – [(2x + 1)/3(x2 + x + 1)]
Practice Problems
Evaluate the following using the method of partial fractions.
- \(\frac{3x}{(x – 1)(x + 2)}\)
- \(\frac{9x^2+5x-3}{(x + 1)^2(x – 2)}\)
- \(\frac{x^2 + 2x – 1}{x(x^2 – 1)}\)
Frequently Asked Questions (FAQs) on Partial Fractions
What is meant by Partial Fractions?
In mathematics, the partial fraction is defined as the process of decomposition of a fraction into the simplest form of the fraction.
Write down the procedure for partial fraction decomposition.
The procedure for the partial fraction decomposition is as follows:
In a given rational expression, factor the denominator into the linear factors
For each factor obtained, write down the partial fraction with variables in the numerator, say x and y
To remove the fraction, multiply the whole equation by the denominator factor.
Now, solve for the constants x, and y
Substitute the constant values in the numerators of the partial fraction, and you will get the solution.
What are the different denominator types in partial fractions?
The four different types of denominator found in the partial fractions are:
Linear factors
Repeated linear factors
Irreducible factors of degree 2
Repeated irreducible factors of degree 2
What is the use of partial fraction decomposition?
Partial fraction decomposition is used to find the inverse Laplace transformation, and also it helps to integrate the rational functions.
What is meant by proper and improper rational expressions?
In proper rational expression, the degree of the numerator is less than the degree of the denominator. Whereas in improper rational expression, the degree of the numerator is greater than the degree of the denominator.
Learn more about Calculus, Integration, Integration by parts, etc. only with BYJU’S – The Learning App and also watch other engaging videos to learn with ease.