Triangular Prism
A triangular prism is a polyhedron, (three-dimensional shape) made up of two triangular bases and three rectangular sides. Like other Prisms, the two bases here are parallel and congruent to each other. It has 5 faces, 6 vertices and 9 edges in total.
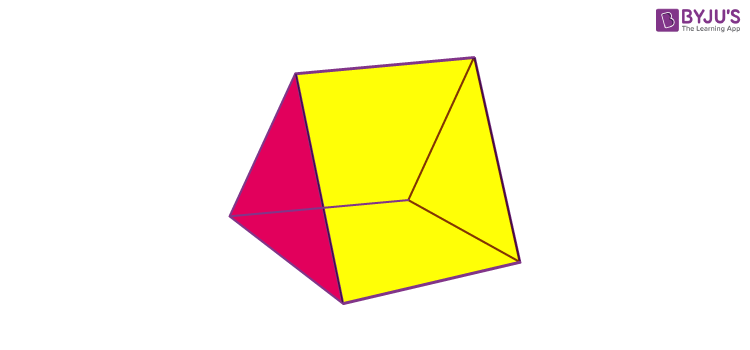
Triangular Prism is a pentahedron and has nine distinct nets. The edges and vertices of the bases are joined with each other via three rectangular sides.
Facts:
|
The sides of triangular prism, which are rectangular in shape are joint with each other side by side. All cross-sections parallel to the base faces are the same as a triangle. A triangular pyramid has four triangular bases unlike the triangular prism, joined with each other and all are congruent to each other.
| Table of contents: |
Check:
Definition
In geometry, a triangular prism is a type of prism with three sides and two bases. The sides are of rectangle shape and bases are of triangle shape. Altogether, it has five faces, nine vertices and six edges.
The sides and bases of the triangular prism are congruent or else oblique. The edges of the prism join the corresponding sides. The two bases of this prism are equilateral triangles and edges of these triangles are parallel to each other. See the below figure to understand the structure.
Other Types of Prisms
Right Triangular Prism
A right triangular prism has its three rectangular sides congruent. Also, the two triangular bases are parallel and congruent to each other. The rectangular or lateral faces are perpendicular to the triangular bases.
Volume
The volume of a triangular prism is equal to the product of the triangular base area and the height of the prism.
| Volume = Area of the Base × Height of prism |
Since, the base is in triangular shape, therefore,
Area = ½ b h
Hence,
| Volume of Triangular Prism = ½ × b × h × l |
Where b is the base length, h is the height of the triangle and l is the length between the triangular bases.
Surface Area
Surface area of triangular prism is equal to the sum of the lateral surface area and twice the base area of the triangular prism. It is measured in square units.
| Surface area of triangular prism = 2A + PH |
Where,
- A is the area of the triangular bases,
- P is the perimeter of the bases and
- H is the height of the prism
Now, Area of the triangular base= ½ × b × h
If a, b and c are the sides of the triangular bases, then,
Perimeter of the base = a + b + c
Therefore,
Surface area of triangular prism = 2(½ × b × h) + ( a + b + c)H
| Surface Area of the Triangular Prism = (bh + ( a + b + c)H) |
Where b and h is the base and height of the bases, respectively and H is the height of the prism.
Properties
Let us discuss some of the properties of the triangular prism.
- It has a total of 9 edges, 5 faces, and 6 vertices(which are joined by the rectangular faces).
- It has two triangular bases and three rectangular sides.
- If the triangular bases are equilateral and the other faces are squares, instead of a rectangle, then the triangular prism is said to be semiregular.
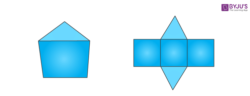
Triangular Prism Net
If we open each face of the triangular prism, we will get the net. The net of this prism comprises three rectangles and two triangles. In the below figure you can see the nine distinct nets.
Solved Examples
Example 1: Find the volume of the triangular prism with base is 5 cm, height is 10 cm, and length is 15 cm.
Solution: Volume of Triangular Prism = ½ × b × h × l
V = ½ × 5 × 10 × 15
Volume, V = 375 cm3
Example 2: If the height of the prism is 4cm and the length of the side of the equilateral triangular base is 6cm. Then find the area of the prism for the above example.
Solutions: We have,
Base = 5cm, height of the base= 10cm, length of the base=15cm, Height of the prism = 4cm
As the base is an equilateral triangle, therefore all its sides will be equal.
Hence, a = b = c = 6cm.
By the formula,
Area of the Triangular Prism = (bh + ( a + b + c)H)
Area = (5 × 10+(6+6+6)4)
=(50 + (18)4) = 50 + 72
= 122 cm2
Download BYJU’S App and subscribe with us to get personalised videos and have fun learning.
Frequently Asked Questions – FAQs
What is a triangular prism?
How many faces, edges and vertices a triangular prism has?
What is the formula to find the volume of a triangular prism?
Volume = ½ × b × h × l
What is the surface area of a triangular prism?
Surface area = bh + ( a + b + c)H
Where a,b and c are the sides of triangular bases and H is the height of the prism.
