Value of Cos 180
The value of cos 180 is equal to -1.
Trigonometry is the study of measurements of triangles which deals with the length, height and angles of the triangle. The trigonometric functions have an enormous application in the real world. In various fields like engineering, architecture, satellite navigation, medical imaging and the development of sound waves etc.
The functions of trigonometry are widely used by engineers, architects, astronauts to calculate the various measurements that undergo the triangular properties. In some applications, it uses the wave pattern of trigonometric functions to produce the sound and light waves. For the creation of computer music, it uses the wave pattern of sine and cosine function. In this article, let us discuss the value of cos 180 or the value of cos pi in detail.
What is the Value of Cos Pi (180°)?
| Value of Cos 180 Degree (π) is -1 |
How to derive the value of Cos 180 Degrees?
The value of cos 180 degrees or the value of cos pi can be represented in terms of different angles like 0°, 90°and 270°.
Consider the unit circle in which the Cartesian plane is divided into four quadrants. To find the value of cos 180 degrees from the Cartesian plane, the value 180 degree takes place in the second quadrant. As the cosine values in the second quadrant always take a negative value.
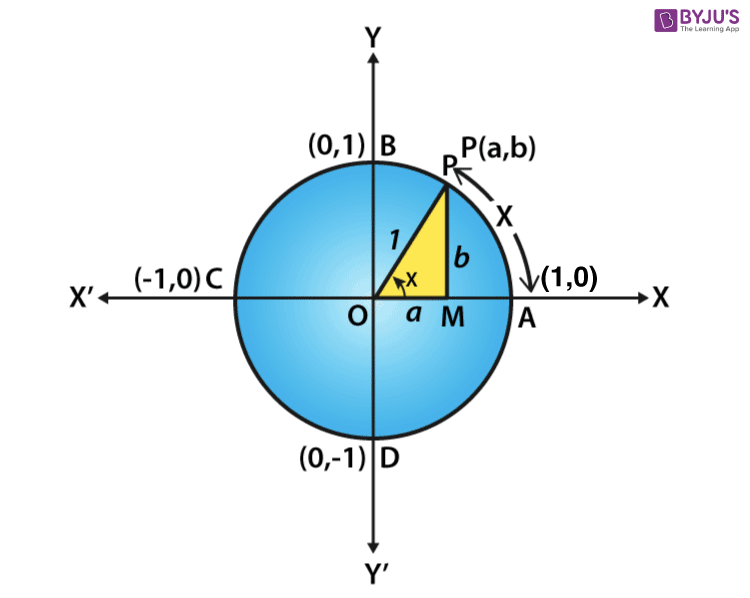
From the value of cos 0, we will obtain the value of cos 180°.
We know that the exact value of cos 0 degrees is 1
So, cos 180 degree is -(cos 0) which is equal to -(1)
Therefore, the value of cos 180 degrees = -1
It is also represented in terms of radians.
So, value of cos pi = -1
There are some other alternative methods to find the value of cos 180°. To find out the value, some degree values of sine functions and cosine functions are taken from the trigonometry table.
We know that,
180° – 0° = 180° ———– (1)
270° – 90° = 180° ———— (2)
Cosine 180 Degree Derivation: Method 1
Now use above representation in cosine function relations, we get
The value of cos pi =Cos 180° = cos(180° – 0°) = – cos 0° = – (1) = – 1
Hence, the value of cos pi = -1
Cosine Pi Value Derivation: Method 2
Cos 180°= cos(270° – 90°)
Cos 180°= – sin 90° (We know that cos ( 270° – a ) = – sin a)
The value of sin 90 degree is 1.
Substitute the value in the above relation, we get
Cos 180°= – (1) = -1
Therefore, the value of cos 180° is -1.
Cos 180°= -1
The value of trigonometric ratios for different angles are given for the reference.
| Trigonometry Ratio Table | ||||||||
|---|---|---|---|---|---|---|---|---|
| Angles (In Degrees) | 0 | 30 | 45 | 60 | 90 | 180 | 270 | 360 |
| Angles (In Radians) | 0 | π/6 | π/4 | π/3 | π/2 | π | 3π/2 | 2π |
| sin | 0 | 1/2 | 1/√2 | √3/2 | 1 | 0 | −1 | 0 |
| cos | 1 | √3/2 | 1/√2 | 1/2 | 0 | −1 | 0 | 1 |
| tan | 0 | 1/√3 | 1 | √3 | Not Defined | 0 | Not Defined | 0 |
| cot | Not Defined | √3 | 1 | 1/√3 | 0 | Not Defined | 0 | Not Defined |
| cosec | Not Defined | 2 | √2 | 2/√3 | 1 | Not Defined | −1 | Not Defined |
| sec | 1 | 2/√3 | √2 | 2 | Not Defined | −1 | Not Defined | 1 |
Visit BYJU’S – The Learning App to learn the values of other trigonometric ratios and concepts and also watch other maths-related videos to learn with ease.
| Related Links | |
| Sin 30 degrees | Cos 0 |
| Cos 90 Degrees | Sine Function |
| Cos Law | Inverse Cosine |