One variable linear equation refers to an equation with only one unknown number, the highest degree of which is 1 and both sides are integers. There is only one root for a linear equation of one variable. Univariate linear equation can solve the vast majority of engineering problems, travel problems, distribution problems, profit and loss problems, integral table problems, telephone billing problems, digital problems.

The monadic equation of degree was first found in ancient Egypt around 1600 BC. Around 820 ad, mathematicians Hua La Zi mi In the book "cancellation and reduction", the idea of "merging similar terms" and "shifting terms" is put forward. In the 16th century, Weida, a mathematician, founded symbols Algebra After that, the propositions of shift and division are put forward . 

Tracing the source of history

The equation of degree of one variable was first seen around 1600 BC Ancient Egypt Period. 

Indian mathematician in the 12th century Pashkaro In the book "lilavatti", we use the hypothesis method (let the unknown number) to solve a class of one variable linear equation. Because the assumed number can be any positive number, boshgaro called the above method "arbitrary number algorithm".

In the 16th century, Veda Founding symbol Algebra After that, he put forward the proposition of the shift term and the same division of the equation, and also created this concept, which is honored as "the father of modern mathematics". But Veda didn't accept negative numbers.

Concept definition

It contains only one unknown number, and the height of the unknown number frequency The equation is called linear equation with one unknown.  Its general form is as follow:

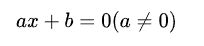
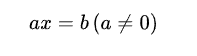
Solving Linear Equation in One Unknown

Consolidation of similar items

The same as what we learned in integral addition and subtraction, the process of merging the terms containing unknowns and constant terms on the same side of the equal sign into one term is called merging congeners. The purpose of merging similar terms is to deform to the form close to x = A and further find the solution of the univariate linear equation.

term shifting

1: Concept: shifting a term on one side of an equation to the other side is called term shifting.

2: Basis: the basis of the transfer term is the property 1 of the equation.

3: Goal: usually move the items with unknowns to the left of the equal sign, and move the items without unknowns to the right of the equal sign, so as to make the equation closer to the form of x = a.

 The coefficient is reduced to 1

1: Concept: transforming the equation in the form of AX = B (a ≠ 0) into the form of x = B / A, that is, the process of finding the solution of the equation x = B / A is called coefficient to 1.

2: Basis: using the property 2 of the equation, the left and right sides of the equation are multiplied by the reciprocal of the unknown coefficient at the same time.

Remove brackets

In the process of solving the equation, the process of removing the brackets contained in the equation is called removing the brackets.

De denominator

1:De denominator method: each term of the unary primary equation is multiplied by the least common multiple of all denominators, and the denominator in the equation becomes 1 according to the property 2 of the equation.

2:The basis for denominator removal: it is the property 2 of the equation, that is, multiply both sides of the equation by the least common multiple of all denominators to convert the coefficients of the equation into integers.

Application of univariate linear equation

linear equation with one unknown can solve most engineering problems, travel problems, distribution problems, profit and loss problems, integral table problems, telephone billing problems and digital problems. If only arithmetic is used, some problems may be extremely complex and difficult to understand. The establishment of the univariate linear equation model will be able to find the equivalent relationship from the actual problems and abstract it into a mathematical problem that can be solved by the univariate linear equation. For example, in the Diophantine problem, it may not be possible to use only the integer, and finding the "age" as an equivalent relationship through the univariate linear equation will simplify the problem. Univariate linear equations can also play a role in the proof of mathematical theorems, such as proving that "the cycle of 0.9 is equal to 1" in the scope of elementary mathematics. By verifying the rationality of the solution of the univariate first-order equation, the purpose of explaining and solving life problems is achieved, and some problems in production and life are solved to a certain extent.