Class 9 Maths Chapter 10 Circles MCQs
Class 9 Maths Chapter 10 Circles MCQs are available online, here with answers. These are the objective questions prepared, as per the CBSE syllabus and NCERT curriculum. The multiple-choice questions are given here chapter-wise, with detailed explanations. Also, check Important Questions for Class 9 Maths.
MCQs on Class 9 Maths Chapter 10 Circles
Solve the MCQs on circles given here with four multiple options and choose the right answer.
1) The center of the circle lies in______ of the circle.
a. Interior
b. Exterior
c. Circumference
d. None of the above
Answer: a
2) The longest chord of the circle is:
a. Radius
b. Arc
c. Diameter
d. Segment
Answer: c
3) Equal _____ of the congruent circles subtend equal angles at the centers.
a. Segments
b. Radii
c. Arcs
d. Chords
Answer: d
Explanation: See the figure below:
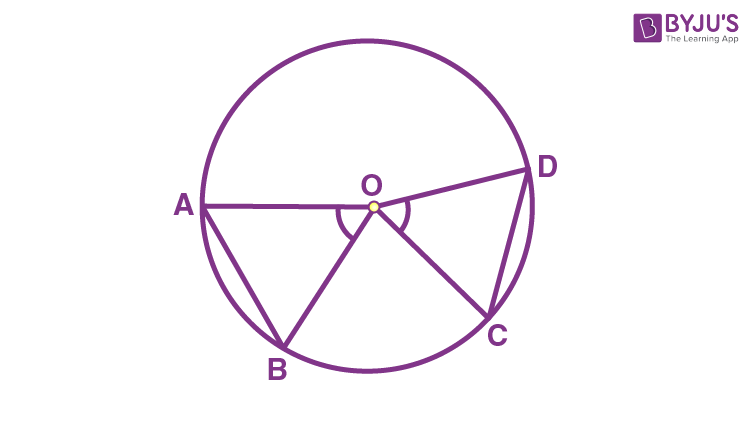
Let ΔAOB and ΔCOD are two triangles inside the circle.
OA = OC and OB = OD (radii of the circle)
AB = CD (Given)
So, ΔAOB ≅ ΔCOD (SSS congruency)
∴ By CPCT rule, ∠AOB = ∠COD.
Hence, this prove the statement.
4) If chords AB and CD of congruent circles subtend equal angles at their centres, then:
a. AB = CD
b. AB > CD
c. AB < AD
d . None of the above
Answer: a
Explanation: Take the reference of the figure from above question.
In triangles AOB and COD,
∠AOB = ∠COD (given)
OA = OC and OB = OD (radii of the circle)
So, ΔAOB ≅ ΔCOD. (SAS congruency)
∴ AB = CD (By CPCT)
5) If there are two separate circles drawn apart from each other, then the maximum number of common points they have:
a. 0
b. 1
c. 2
d. 3
Answer: a
6) The angle subtended by the diameter of a semi-circle is:
a. 90
b. 45
c. 180
d. 60
Answer: c
Explanation: The semicircle is half of the circle, hence the diameter of the semicircle will be a straight line subtending 180 degrees.
7) If AB and CD are two chords of a circle intersecting at point E, as per the given figure. Then:

a.∠BEQ > ∠CEQ
b. ∠BEQ = ∠CEQ
c. ∠BEQ < ∠CEQ
d. None of the above
Answer: b
Explanation:
OM = ON (Equal chords are always equidistant from the centre)
OE = OE (Common)
∠OME = ∠ONE (perpendiculars)
So, ΔOEM ≅ ΔOEN (by RHS similarity criterion)
Hence, ∠MEO = ∠NEO (by CPCT rule)
∴ ∠BEQ = ∠CEQ
8) If a line intersects two concentric circles with centre O at A, B, C and D, then:
a. AB = CD
b. AB > CD
c. AB < CD
d. None of the above
Answer: a
Explanation: See the figure below:

From the above fig., OM ⊥ AD.
Therefore, AM = MD — 1
Also, since OM ⊥ BC, OM bisects BC.
Therefore, BM = MC — 2
From equation 1 and equation 2.
AM – BM = MD – MC
∴ AB = CD
9) In the below figure, the value of ∠ADC is:
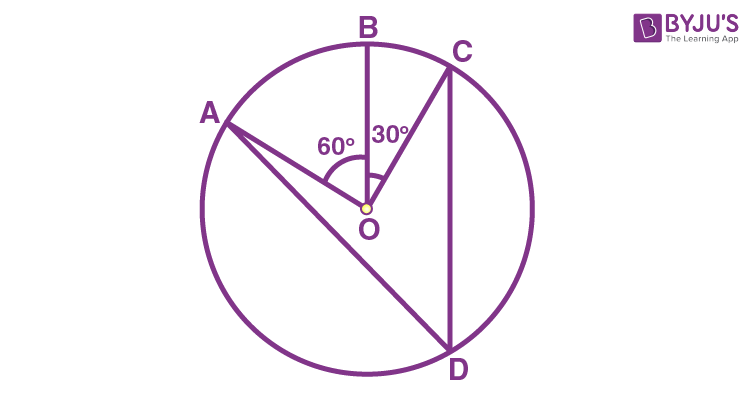
a. 60°
b. 30°
c. 45°
d. 55°
Answer: c
Explanation: ∠AOC = ∠AOB + ∠BOC
So, ∠AOC = 60° + 30°
∴ ∠AOC = 90°
An angle subtended by an arc at the centre of the circle is twice the angle subtended by that arc at any point on the rest part of the circle.
So,
∠ADC = 1/2∠AOC
= 1/2 × 90° = 45°
10) In the given figure, find angle OPR.
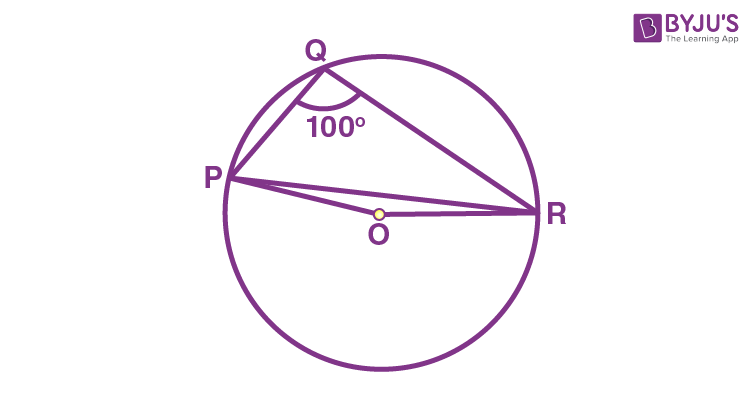
a. 20°
b. 15°
c. 12°
d. 10°
Answer: d
Explanation: The angle subtended by major arc PR at the centre of the circle is twice the angle subtended by that arc at point, Q, on the circle.
So, ∠POR = 2 × ∠PQR, here ∠POR is the exterior angle
We know the values of angle PQR as 100°
So, ∠POR = 2 × 100° = 200°
∴ ∠ROP = 360° – 200° = 160° [Full rotation: 360°]
Now, in ΔOPR,
OP and OR are the radii of the circle
So, OP = OR
Also, ∠OPR = ∠ORP
By angle sum property of triangle, we know:
∠ROP + ∠OPR + ∠ORP = 180°
∠OPR + ∠OPR = 180° – 160°
As, ∠OPR = ∠ORP
2∠OPR = 20°
Thus, ∠OPR = 10°
11) In the given figure, ∠AOB = 90º and ∠ABC = 30º, then ∠CAO is equal to:

(a) 30º
(b) 45º
(c) 60º
(d) 90º
Answer: c
Explanation:
Given that ∠AOB = 90º and ∠ABC = 30º
OA = OB (Radii of the circle)
Let x= ∠OAB = ∠OBA = x
In the triangle OAB,
∠OAB + ∠OBA + ∠AOB = 180° (By using the angle sum property of triangle)
⇒ x + x + 90° = 180°
⇒ 2x = 180° – 90°
⇒ x = 90°/ 2 = 45°
Therefore, ∠OAB = 45° and ∠OBA = 45°
By using the theorem, “ the angles subtended by arcs at the centre of the circle double the angle subtended at the remaining part of the circle”, we can write
∠AOB = 2∠ACB
This can also be written as,
∠ACB = ½ ∠AOB = (½) × 90° = 45°
Now, apply the angle sum property of triangle on the triangle ABC,
∠ACB + ∠BAC + ∠CBA = 180°
∠ACB + [∠BAO + ∠CAO] + ∠CBA = 180° (As,∠BAC = ∠BAO + ∠CAO)
Now, substitute the known values, we get
45° + (45° + ∠CAO) + 30° = 180°
∠CAO = 180°- (30° + 45° + 45°)
∠CAO = 180°-120°
∠CAO = 60°
Hence, ∠CAO is equal to 60°.
12) ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and ∠ADC = 140º, then ∠BAC is equal to:
(a) 30º
(b) 40º
(c) 50º
(d) 80º
Answer: c
Explanation:
Given that ABCD is a cyclic quadrilateral and ∠ADC = 140º.
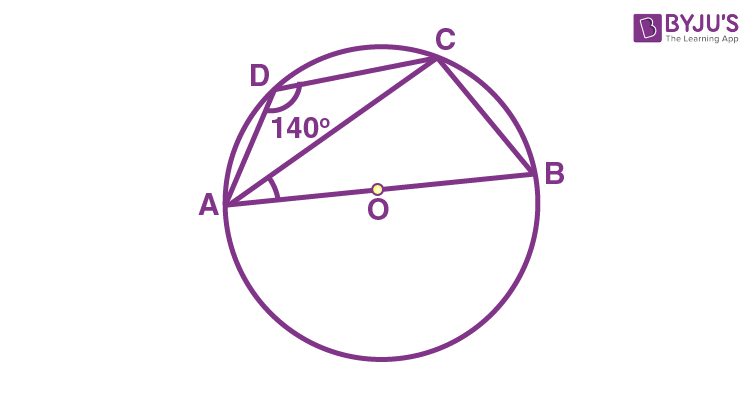
We know that the sum of opposite angles of a cyclic quadrilateral is 180°.
Hence, ∠ADC + ∠ABC = 180°
Now, substitute ∠ADC = 140º in the above equation, we get
140° + ∠ABC = 180°
∠ABC = 180° – 140° = 40°
since the angle subtended by a diameter at the circumference of the circle, is 90°
Hence, ∠ACB = 90°
By using the angle property of triangle in the triangle, ABC,
∠CAB + ∠ABC + ∠ACB = 180°
∠CAB + 40° + 90° = 180°
∠CAB = 180° – 90° – 40°
∠CAB = 50°
Therefore, ∠CAB or ∠BAC =50°.
13) In the given figure, if ∠OAB = 40º, then ∠ACB is equal to

(a) 40º
(b) 50º
(c) 60º
(d) 70º
Answer: b
Explanation:
Given that ∠OAB = 40º,
In the triangle OAB,
OA = OB (radii)
Since, the angles opposite to equal sides are equal,
∠OAB = ∠OBA (i.e.) ∠OBA = 40°
Now, by using the angle sum property of triangle, we can write
∠AOB + ∠OBA + ∠BAO = 180°
Now, substitute the known values,
∠AOB + 40° + 40° = 180°
∠AOB = 180 – 80° = 100°
∠AOB = 2 ∠ACB (Since, the angle subtended by an arc at the centre is twice the angle subtended by it at the remaining part of the circle)
∠ACB = ½ ∠AOB
Hence, ∠ACB = 100°/2 = 50°.
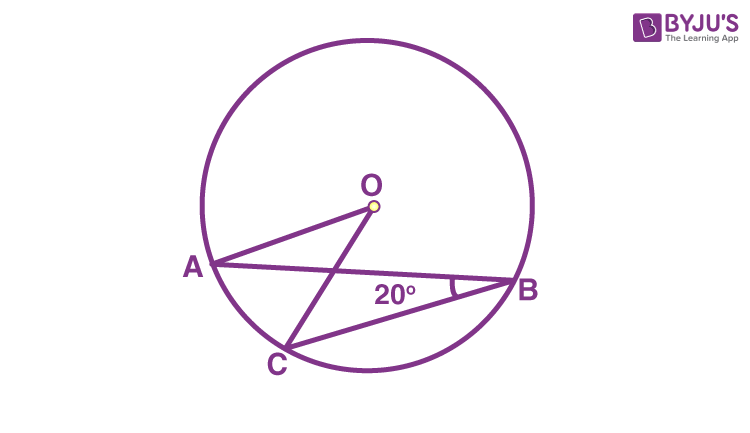
14) In the given figure, if ∠ABC = 20º, then ∠AOC is equal to:
(a) 10º
(b) 20º
(c) 40º
(d) 60º
Answer: c
Explanation:
Given that, ∠ABC = 20º.
∠AOC = 2∠ABC (since the angle subtended by an arc at the centre of the circle is double the angle subtended at the remaining part.)
Now, substitute the values, we get
∠AOC = 2 × 20°
Therefore, ∠AOC = 40°.
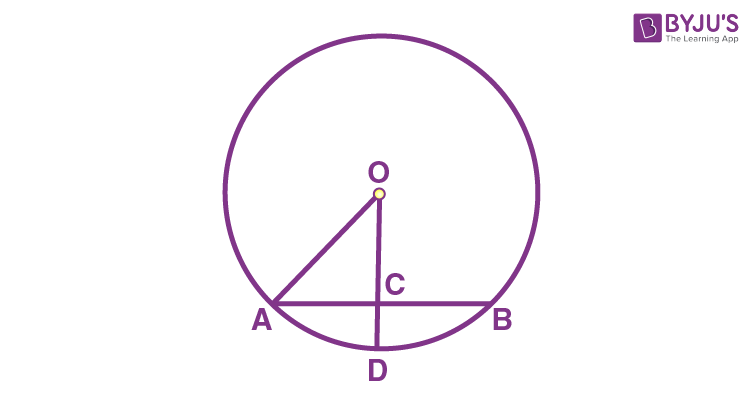
15) In the given figure, if OA = 5 cm, AB = 8 cm and OD is perpendicular to AB, then CD is equal to:
(a) 2 cm
(b) 3 cm
(c) 4 cm
(d) 5 cm
Answer: a
Explanation:
From the given diagram, we can observe that OC is perpendicular to chord AB. Therefore, OC bisects the chord AB Hence. AC=CB
Also,
AC+CB=AB
AC+CB=8
2AC = 8 (Since, AC = CB)
AC = 8/2 = 4 cm
As, the triangle OCA is a right-angled triangle, by using Pythagoras theorem, we can write
AO2=AC2+OC2
52=42+OC2
52−42=OC2
OC2=9
OC=3 cm
As, OD is the radius of the circle, OA=OD=5cm
CD=OD-OC
CD = 5-3 = 2 cm
Hence, the value of CD is equal to 2cm.
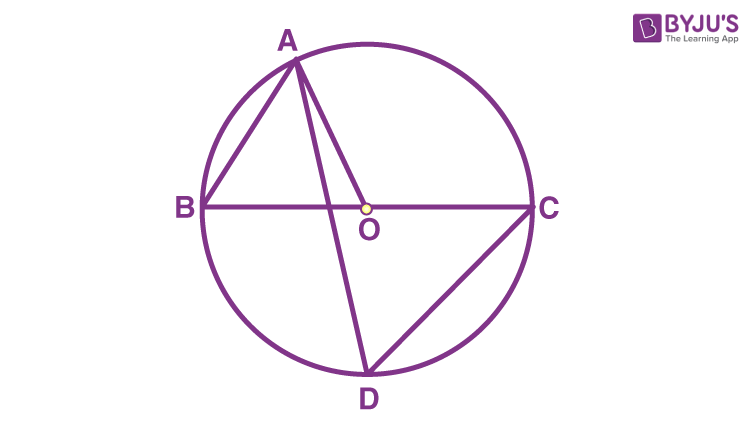
16) In the given figure, BC is the diameter of the circle and ∠BAO = 60º. Then ∠ADC is equal to
(a) 30º
(b) 45º
(c) 60º
(d) 120º
Answer: c
Explanation:
Given that ∠BAO = 60°
Since OA = OB,∠OBA = 60°
Then ∠ADC = 60° (As, the angles in the same segment are equal).
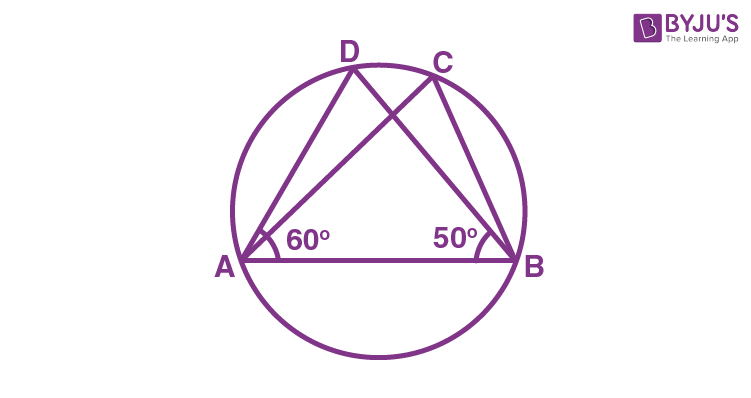
17) In the given figure, if ∠DAB = 60º, ∠ABD = 50º, then ∠ACB is equal to:
(a) 50º
(b) 60º
(c) 70º
(d) 80º
Answer: c
Explanation:
Given that, ∠DAB = 60º, ∠ABD = 50º
By using the angle sum property in the triangle ABD
∠DAB+∠ABD+∠ADB=180º
60º+50º+∠ADB=180º
∠ADB=180º-110º
∠ADB=70º
∠ADB=∠ACB (Since the angles subtended at the circumference by the same arc are equal)
We know that the angles subtended at the circumference by the same arc are equal.
∠ACB =70º
18) In the given figure, if AOB is a diameter of the circle and AC = BC, then ∠CAB is equal to:

(a) 30º
(b) 45º
(c) 60º
(d) 90º
Answer: b
Explanation:
We know that the angle at circumference subtended by the diameter of the circle is the right angle.
Hence, ∠ACB = 90°
Also, given that AC = BC
Therefore, ∠CAB = ∠CBA (As, the angles opposite to equal sides are also equal)
Now, by using the angle sum property of triangle in ∆ACB, we can write
∠CAB + ∠ABC + ∠BCA = 180°
∠CAB + ∠CAB + 90° = 180°
2∠CAB = 180° – 90°
∠CAB = 45°
Therefore, ∠CAB is equal to 45°.
19) If AB = 12 cm, BC = 16 cm and AB is perpendicular to BC, then the radius of the circle passing through the points A, B and C is:
(a) 6 cm
(b) 8 cm
(c) 10 cm
(d) 12 cm
Answer: c
Explanation:
Given that AB = 12 cm, BC = 16 cm and AB is perpendicular to BC.

Hence, AC is the diameter of the circle passing through points A, B and C.
Hence, ABC is a right-angled triangle.
Thus by using the Pythagoras theorem:
AC2 = (CB)2 + (AB)2
⇒ AC2 = (16)2 + (12)2
⇒ AC2 = 256 + 144
⇒ AC2 = 400
Hence, the diameter of the circle, AC = 20 cm.
Thus, the radius of the circle is 10 cm.
20) AD is the diameter of a circle and AB is a chord. If AD = 34 cm, AB = 30 cm, the distance of AB from the centre of the circle is
(a) 4 cm
(b) 8 cm
(c) 15 cm
(d) 17 cm
Answer: b
Explanation:
Given that, Diameter, AD = 34 cm.
Chord, AB = 30 cm.

Hence, the radius of the circle, OA = 17 cm
Now, consider the figure.
From the centre “O”. OM is perpendicular to the chord AB.
(i.e) OM ⊥ AM
AM = ½ AB
AM = ½ (30) = 15 cm
Now by using the Pythagoras theorem in the right triangle AOM,
AO2 = OM2 + AM2
OM2 = AO2– AM2
OM2= 172 – 152
OM2 = 64
OM = √64
OM = 8 cm
Stay tuned with BYJU’S – The Learning App and download the app today to get more class-wise concepts.