Class 10 Maths Chapter 7 Coordinate Geometry Notes

The complete notes on coordinate geometry class 10 are provided here. Go through the below article and learn the points on the coordinate plane, distance formulas, section formulas and so on with a detailed explanation.

Students can refer to the short notes and MCQ questions along with separate solution pdf of this chapter for quick revision from the links below:

• Coordinate Geometry Short Notes
• Coordinate Geometry MCQ Practice Questions
• Coordinate Geometry MCQ Practice Solutions

Basics of Coordinate Geometry

For More Information On Basics of Coordinate Geometry, Watch The Below Video.

To know more about Coordinate Geometry, visit here.

Points on a Cartesian Plane

A pair of numbers locate points on a plane called the coordinates. The distance of a point from the y-axis is known as abscissa or x-coordinate. The distance of a point from the x-axis is called ordinates or y-coordinate.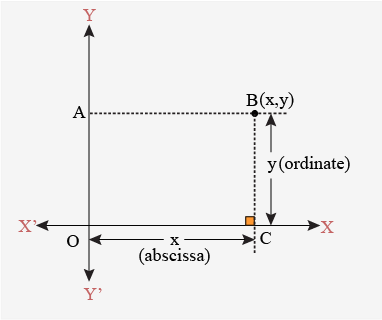

Distance Formula

Distance between Two Points on the Same Coordinate Axes

The distance between two points that are on the same axis (x-axis or y-axis), is given by the difference between their ordinates if they are on the y-axis, else by the difference between their abscissa if they are on the x-axis.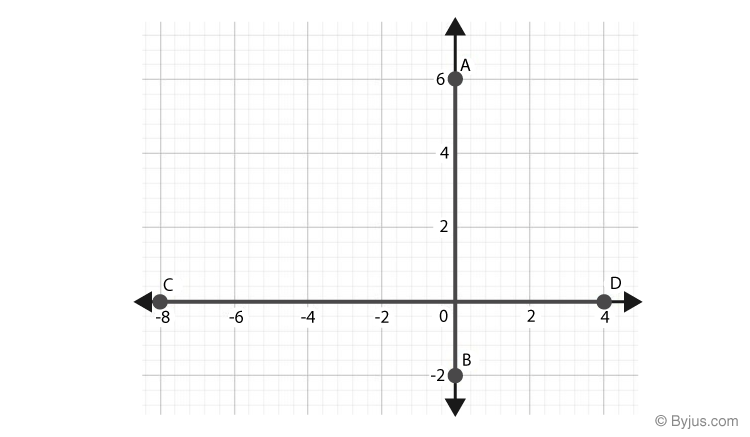

Distance AB = 6 – (-2) = 8 units

Distance CD = 4 – (-8) = 12 units

Distance between Two Points Using Pythagoras Theorem

 

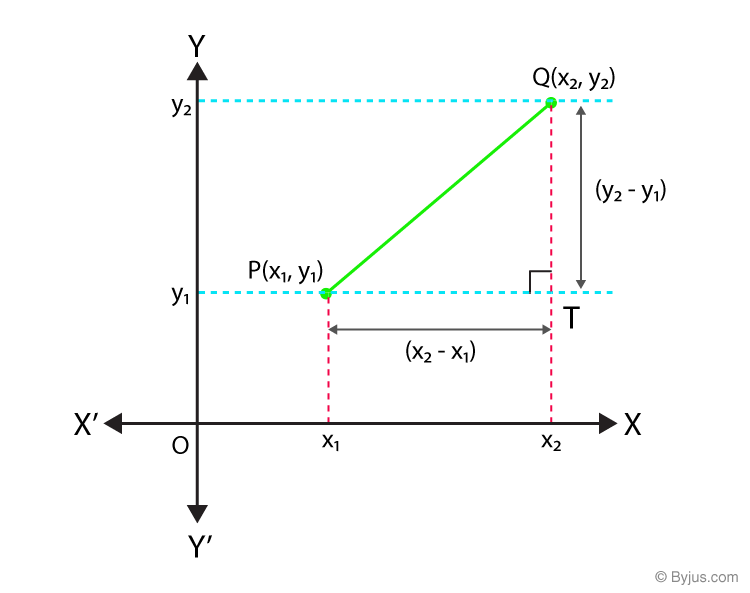
Let P(x₁, y₁) and Q(x₂, y₂) be any two points on the cartesian plane.

Draw lines parallel to the axes through P and Q to meet at T.

ΔPTQ is right-angled at T.

By Pythagoras Theorem,

PQ² = PT² + QT²

= (x₂ – x₁)² + (y₂ – y₁)²

PQ = √[x₂ – x₁)² + (y₂ – y₁)²]

Distance Formula

Distance between any two points (x₁, y₁) and (x₂, y₂) is given by

d = √[x₂ – x₁)²+(y₂ – y₁)²]

Where d is the distance between the points (x₁,y₁) and (x₂,y₂).

To know more about Distance Formula, visit here.

Section Formula

If the point P(x, y) divides the line segment joining A(x₁, y₁) and B(x₂, y₂) internally in the ratio m:n, then, the coordinates of P are given by the section formula as:

\(P(x,\ y)=(\frac{mx_{2}+nx_{1}}{m+n},\frac{my_{2}+ny_{1}}{m+n} )\)

For More Information On Section Formula, Watch The Below Video.

To know more about Section Formula, visit here.

Finding ratio given the points

To find the ratio in which a given point P(x, y) divides the line segment joining A(x₁, y₁) and B(x₂, y₂),

• Assume that the ratio is k : 1
• Substitute the ratio in the section formula for any of the coordinates to get the value of k.

\(x=\frac{kx_{2}+x_{1}}{k+1}\)

When x1, x2 and x are known, k can be calculated. The same can be calculated from the y- coordinate also.

MidPoint

The midpoint of any line segment divides it in the ratio 1 : 1.

The coordinates of the midpoint(P) of line segment joining A(x₁, y₁) and B(x₂, y₂) is given by

\(p(x,\ y)=(\frac{x_{1}+x_{2}}{2},\frac{y_{1}+y_{2}}{2} )\)

Points of Trisection

To find the points of trisection P and Q which divides the line segment joining A(x₁, y₁) and B(x₂, y₂) into three equal parts:

i) AP : PB = 1 : 2

\(P=(\frac{x_{2}+2x_{1}}{3},\frac{y_{2}+2y_{1}}{3} )\)

ii) AQ : QB = 2 : 1

\(Q=(\frac{2x_{2}+x_{1}}{3},\frac{2y_{2}+y_{1}}{3} )\)

Centroid of a triangle

If A(x₁, y₁), B(x₂, y₂) and C(x₃, y₃) are the vertices of a ΔABC, then the coordinates of its centroid(P) is given by

\(p(x,\ y)=(\frac{x_{1}+x_{2}+x_{3}}{3},\frac{y_{1}+y_{2}+y_{3}}{3} )\)

Area from Coordinates

Area of a triangle given its vertices

If A(x₁, y₁),B(x₂, y₂) and C(x₃, y₃) are the vertices of a Δ ABC, then its area is given by

A = (1/2)[x₁(y₂ − y₃) + x₂(y₃ − y₁) + x₃(y₁ − y₂)]

Where A is the area of the Δ ABC.

To know more about Area of a Triangle, visit here.

Collinearity Condition

If three points A, B and C are collinear and B lies between A and C, then,

• AB + BC = AC. AB, BC, and AC can be calculated using the distance formula.
• The ratio in which B divides AC, calculated using section formula for both the x and y coordinates separately will be equal.
• Area of a triangle formed by three collinear points is zero.

Coordinate Geometry for Class 10 Problems

Example 1:

Determine the distance between the pair of points (a, b) and (-a, -b)

Solution:

Let the given points be A(a, b) and B(-a, -b)

We know that the distance formula is:

AB = √[(x₂-x₁)²+(y₂-y₁)²]

(x₁, y₁) = (a, b)

(x₂, y₂) = (-a, -b)

Now, substitute the values in the distance formula, we get

AB = √[(-a-a)²+(-b-b)²]

AB = √[(-2a)² + (-2b)²]

AB = √[4a²+4b²]

AB = √[4(a²+b²)]

AB = √4. √[a²+b²]

AB = 2.√[a²+b²].

Hence, the distance between two points (a, b) and (-a, -b) is 2√[a²+b²].

Example 2:

Determine the ratio in which the line segment joining the points A(1, -5) and B(-4, 5) is divided by the x-axis. Also, find the coordinates of the point of division.

Solution:

Given that, the point P is on the x-axis. Hence, y-coordinate is 0. Hence, the point is of the form P(x, 0).

Now, we have to find the ratio. Let the ratio be k:1.

Given Points: A(1, -5) and B = (-4, 5).

(x₁, y₁) = (1, -5)

(x₂, y₂) = (-4, 5)

m₁ = k, m₂ = 1

We know that the section formula is:

y= [m₁y₂+m₂y₁]/[m₁+m₂]

Now, substitute the values in the section formula, we get

y = [k(5) + 1(-5)]/[k+1]

y = [5k-5]/[k+1]

Since, y=0

(5k-5)/(k+1) = 0

5k -5 = 0

5k = 5

k=1

Hence, the ratio k:1 = 1:1

Finding x-coordinate:

x= [m₁x₂+m₂x₁]/[m₁+m₂]

x = [k(-4) + 1(1)]/(k+1)

Now, substitute k=1 in the above equation, we get

x = [1(-4) + 1(1)]/(1+1)

x = (-4+1)/2

x = -3/2.

Hence, the coordinate of point is P(x, 0) = P(-3/2, 0).

Stay tuned with BYJU’S – The Learning App and download the app to learn all Maths-related concepts easily by exploring more videos.