Factors of 81
The factors of 81 are the numbers that produce the result as 81 when two numbers are multiplied together. Consider an example, the factor pair of 7 is written as (1,7) and (-1,-7). When we multiply the pair of negative factors, the result should give the original number, such as multiplying -1 × -7, then we get the number 7. Thus, we can consider both positive and negative factor pairs of 7. Factor pairs of the number 81 are the whole numbers which could be either positive or negative but not a fraction or decimal number. To find the factors of a number, 81, we will use the factorization method.
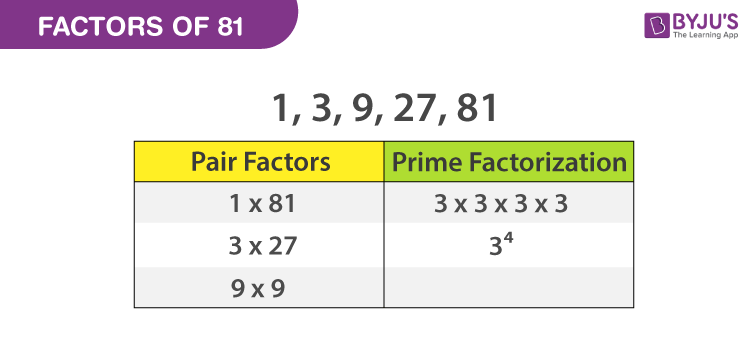
In the factorization method, first, consider the numbers 1 and 81 as factors and continue finding the other pair of factors of 81, which gives the results as an original number. To understand this method better, read the article below to find the factors of 81 in pairs. Also, the prime factors with the help of the division method is explained below.
Important Facts of Factors
- A factor of any number is its exact divisor
- 1 is a factor of every number.
- Any factor of a number is always less than or equal to the number itself
- The number itself is the greatest factor.
Pair Factors of 81
To find the factors of 81 in pairs, multiply the two numbers in a pair to get the original number as 81, such numbers are as follows
If 1 × 81 = 81, then (1, 81) is a pair factor of 81.
Similarly, let us find other pair factors.
3 × 27 = 81, (3, 27) is a pair factor of 81
9 × 9 = 81, (9, 9) is a pair factor of 81
Therefore, the positive pair factors are (1, 81), (3, 27), and (9, 9).
To find the negative pair factors, proceed with the following steps
If -1 × -81 = 81, then (-1,- 81) is a pair factor of 81
-3 × -27 = 81, (-3, -27) is a pair factor of 81
-9 × -9 = 81, (-9, -9) is a pair factor of 81.
Therefore, the negative pair factors are (-1, -81), (-3, -27), and (-9,- 9).
How to calculate the Factors of 81?
Go through the following steps to calculate the factors.
- First, write the number 81
- Find the two numbers, which gives the result as 81 under the multiplication, say 3 and 27, such as 3 × 27 = 81.
- We know that 3 is a prime number which has only two factors, i.e., 1 and the number itself( 1 and 3). So, it cannot be further factorized.
3 = 3 × 1 - Look at the number 27, which is a composite number but not a prime number. So it can be further factorized.
27 = 3 × 3 × 3 × 1 - Therefore, the factorization of 81 is written as 81 = 3 x 3 × 3 × 3 × 1
- Finally, write down all the unique numbers which we can obtain from 3 × 3 × 3 × 3 × 1.
| Factors of 81 |
| 1, 3, 9, 27, and 81 |
Prime Factors of 81 By Division Method
The number 81 is a composite and it must have prime factors. Now let us know how to calculate the prime factors of a number 81.
- The first step is to divide the number 81 with the smallest prime number say 2.
81 ÷ 2 = 40.5, not a factor since it is a decimal number - Now, divide 81 by the next prime number, say 3.
81 ÷ 3 = 27
Again, divide 81 by 3 and the process goes on.
27 ÷ 3 = 9
9 ÷ 3 = 3
3 ÷ 3 = 1
- Finally, we received the number 1 at the end of the division process. So that we cannot proceed further. So, the prime factors are written as 3 × 3 × 3 × 3 or 34, where 3 is a prime number.
Stay tuned with BYJU’S to know more about factors and prime factors of other numbers and also download BYJU’S – The Learning App for a better experience and clarification.
| Links Related to Factors | |
| Factors of 15 | Factors of 36 |
| Factors of 48 | Factors of 18 |
| Factors of 24 | Factors of 25 |
| Factors of 42 | Factors of 60 |
| Factors of 35 | Factors of 27 |
| Factors of 84 | Factors of 56 |
Frequently Asked Questions on Factors of 81 – FAQs
Is 6 a factor of 81 yes or no?
What are the factors of 81 and 90?
The factors of 90 are: 1, 2, 3, 5, 6, 9, 10, 15, 18, 30, 45, and 90.
What is the GCF of 81 and 88?
The prime factorisation of 88 is: 2 × 2 × 2 × 11
What are all the factors of 80?
What are factors of 200?
1, 2, 4, 5, 8, 10, 20, 25, 40, 50, 100 and 200