Perimeter of Rectangle
Perimeter of Rectangle could be considered as one of the important formulae of the rectangle. It is the total distance covered by the rectangle around its outside. In Maths, you will come across many geometric shapes and sizes, which have an area, perimeter and even volume (for 3-d figures). You will also learn the formulas for all those parameters. Some of the examples of different shapes are circle, square, polygon, quadrilateral, etc. In this article, you will study the key feature of the rectangle, i.e. perimeter.
Perimeter basically gives the length of the figure. Suppose for a square, which has all its sides as equal, the perimeter of the square will be four times its sides. In the case of a circle, the perimeter is termed as circumference, which is calculated based on its radius. Before we find out the perimeter of a given rectangle, let us learn first, what a rectangle is.
A rectangle is a quadrilateral that has two pairs of parallel sides equal and all the four angles at the vertices are right angles.
What is Perimeter of Rectangle?
The perimeter of a rectangle is the total distance covered by its boundaries or the sides. Since there are four sides of a rectangle, thus, the perimeter of the rectangle will be the sum of all four sides. Since the perimeter is a linear measure, therefore, the unit of the perimeter of rectangle will be in meters, centimeters, inches, feet, etc.
Perimeter of a Rectangle Formula
The perimeter of a rectangle is defined as the sum of all the sides of a rectangle. For any polygon, the perimeter formulas are the total distance around its sides. In case of a rectangle, the opposite sides of a rectangle are equal and so, the perimeter will be twice the width of the rectangle plus twice the length of the rectangle and it is denoted by the alphabet “p”. Let us derive the formula for its perimeter and area.
Suppose a rectangle has length and width as b and a, respectively.
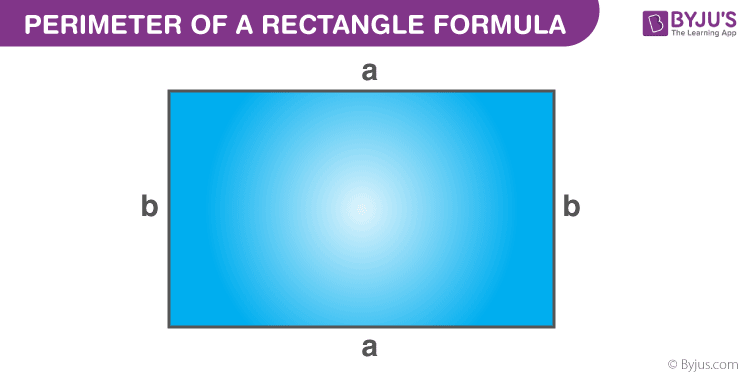
From the definition of the perimeter we know, the perimeter of a rectangle, P = 2 ( a+b) units
where
“a” is the length of the rectangle
“b” is the breadth of the rectangle
Derivation of Perimeter of Rectangle
Since the perimeter is equal to the sum of all the sides of the polygon. Hence, in the case of a rectangle, the perimeter (P) is;
P = sum of all its four sides
P = a + b + a + b (Opposite sides of rectangle are equal)
P = 2(a + b)
Hence, derived.
Therefore,
| Perimeter of a rectangle = 2(Length + Width) square units |
Now let us write the formula for the area of a rectangle, with respect to same above given figure;
| Area of a rectangle = Length × Width = a × b |
Applications of Perimeter of Rectangle
There are many real-life applications of the perimeter of a rectangle. A few of them are listed below:
- We can determine the length of a rectangular field or a garden for its fencing using the perimeter formula
- It can be used for many art and craft projects such as decorating the border of rectangular cardboard with colourful ribbons or ropes
- For the construction of a rectangular swimming pool, the length of swimming races are defined by the perimeter
- For the construction plan of the house, we need to set a boundary using concrete that is possible by perimeter formula
Related Articles
Solved Examples on Perimeter of Rectangle
Q.1: Find the perimeter of a rectangle whose length and width are 5 cm and 10 cm, respectively.
Solution: Given:
Length = 5 cm and Width = 10 cm
We know,
The perimeter of a rectangle = 2(length + width)
Substitute the value of length and width here,
Perimeter, P = 2(5 + 10) cm
P = 2 x 15 cm
Therefore, the perimeter of a rectangle = 30 cm
Q.2: Find the perimeter of a rectangle whose length and breadth are 12 cm and 15 cm, respectively.
Solution:
Given:
Length = 12 cm and Breadth = 15 cm
We know,
The perimeter of a rectangle = 2(length + width)
Substitute the value of length and width here,
Perimeter, P = 2(12 + 15) cm
P = 2 x 27 cm
Therefore, the perimeter of a rectangle = 54 cm
Q.3: A rectangular yard has length equals to 10 cm and perimeter equals to 60 cm. Find its width.
Solution: Given,
Perimeter of the yard = 60 cm
Length of the yard = 10 cm
Let W be the width of the yard.
From the formula, we know,
Perimeter, P = 2(length + width)
Substituting the values, we get;
60 = 2(10 + width)
10 + W = 30
W = 30 – 10 = 20
Hence, the width of the yard is 20cm.
Q.4: Find the perimeter of a rectangle whose length is 9 cm and width is 16 cm.
Solution:
Given,
Length = 9 cm
Width = 16 cm
Perimeter of Rectangle= 2(Length + Width)
= 2(9 + 16) cm
= 2 x 25 cm
Therefore, the perimeter of a rectangle= 50 cm
Q.5: Find the perimeter of a rectangle whose length and width is 20 cm and 9 cm, respectively.
Solution:
Given,
Length = 20 cm
Width = 9 cm
Perimeter of Rectangle = 2(Length + Width)
= 2(20 +9) cm
= 2 x 29 cm
Therefore, the perimeter of a rectangle = 58 cm
To learn about more geometrical concepts, learn with BYJU’S and also download its app to get personalised learning videos.
Practice Questions
- Find the perimeter of a given rectangle which has length = 15 cm and width = 20 cm.
- What is the length of the rectangular field which has a perimeter of 100 cm and width of 25 cm?
Frequently Asked Questions on Perimeter of Rectangle
What is the area and perimeter of a rectangle?
The area of a rectangle can be defined as the region that the rectangle covers in a two-dimensional space. The area of a rectangle can also be defined as the number of square units it takes to completely fill the rectangle.
The perimeter of a rectangle is defined as the total distance around the outside of a rectangle. In simple words, a rectangle’s perimeter is the total boundary of it.
How to find the perimeter of a rectangle?
To get the perimeter of a rectangle, add all the sides of it. There are 4 sides of a rectangle whose sum will give its perimeter.
What is the formula for the perimeter of a rectangle?
The formula for the perimeter of a rectangle is, P = length + breadth + length + breadth.
Or, P = 2(length + breadth)