Period of a Function
The time interval between two waves is known as a Period whereas a function that repeats its values at regular intervals or periods is known as a Periodic Function. In other words, a periodic function is a function that repeats its values after every particular interval.
The period of the function is this particular interval mentioned above.
A function f will be periodic with period m, so if we have
f (a + m) = f (a), For every m > 0.
It shows that the function f(a) possesses the same values after an interval of “m”. One can say that after every interval of “m” the function f repeats all its values.
For example – The sine function i.e. sin a has a period of 2π because 2π is the smallest number for which sin (a + 2π) = sin a, for all a.
We may also calculate the period using the formula derived from the basic sine and cosine equations. The period for function y = A sin(Bx + C) and y = A cos(Bx + C) is 2π/|B| radians.
The reciprocal of the period of a function = frequency
Frequency is defined as the number of cycles completed in one second. If the period of a function is denoted by P and f be its frequency, then –f =1/ P.
Fundamental Period of a Function
The fundamental period of a function is the period of the function which are of the form,
f(x+k)=f(x)
f(x+k)=f(x), then k is called the period of the function and the function f is called a periodic function.
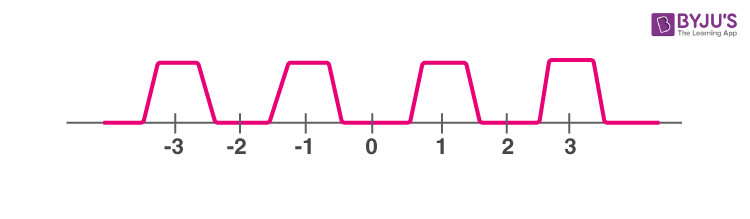
Now, let us define the function h(t) on the interval [0, 2] as follows:

If we extend the function h to all of R by the equation,
h(t+2)=h(t)
=> h is periodic with period 2.
The graph of the function is shown below.
How to Find the Period of a Function?
- If a function repeats over at a constant period we say that is a periodic function.
- It is represented like f(x) = f(x + p), p is the real number and this is the period of the function.
- Period means the time interval between the two occurrences of the wave.
Let’s learn how to fund the period and amplitude of a given trigonometric function, such as sine, cosine, tangent, etc., along with graphs and examples here.
Period of a Trigonometric Function
The distance between the repetition of any function is called the period of the function. For a trigonometric function, the length of one complete cycle is called a period. For any trigonometry graph function, we can take x = 0 as the starting point.
In general, we have three basic trigonometric functions like sin, cos and tan functions, having -2π, 2π and π periods respectively.
Sine and cosine functions have the forms of a periodic wave:
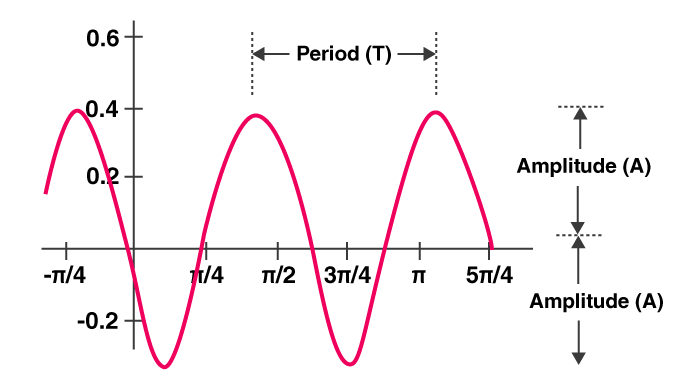
- Period: It is represented as “T”. A period is a distance among two repeating points on the graph function.
- Amplitude: It is represented as “A”. It is the distance between the middle point to the highest or lowest point on the graph function.
sin(aθ) = 2πa and cos(aθ) = 2πa
Period of a Sine Function
If we have a function f(x) = sin (xs), where s > 0, then the graph of the function makes complete cycles between 0 and 2π and each of the function have the period, p = 2π/s
Now, let’s discuss some examples based on sin function:
Let us discuss the graph of y = sin 2x
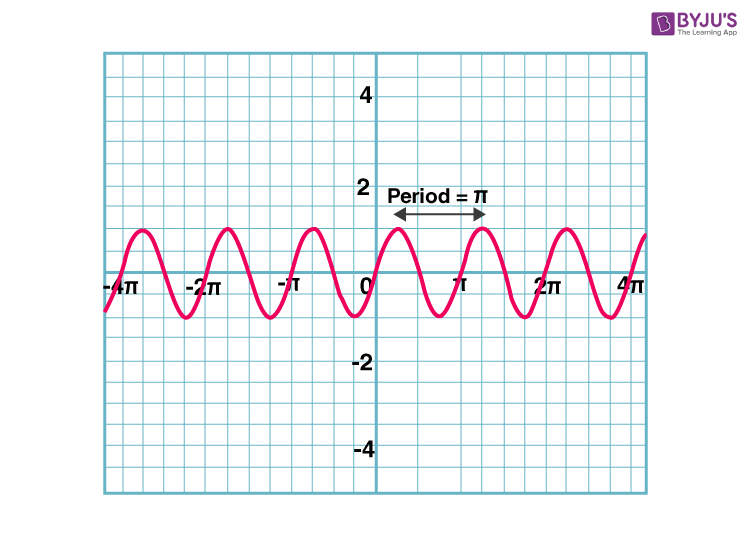
| Period = π | Axis: y = 0 [x-axis ] | Amplitude: 1 | Maximum value = 1 |
| Minimum value = -1 | Domain: { x : x ∈ R } | Range = [ -1, 1] | – |
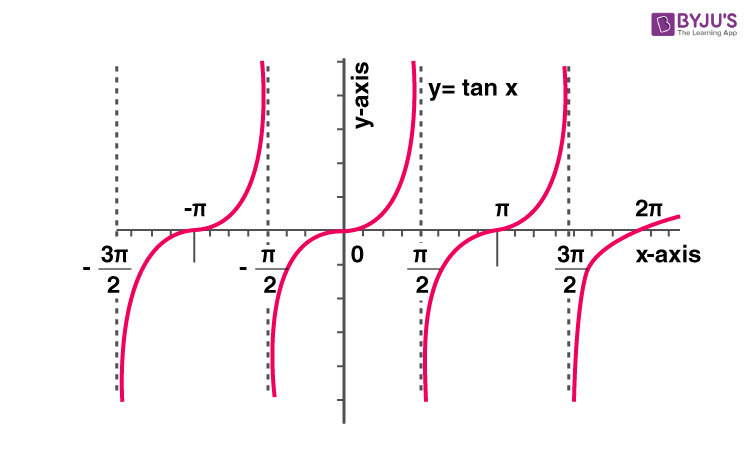
Period of a Tangent Function
If we have a function f(a) = tan (as), where s > 0, then the graph of the function makes complete cycles between −π/2, 0 and π/2 and each of the function have the period of p = π/s
Periodic Functions Examples
Let’s learn some of the examples of periodic functions.
Example 1:
Find the period of the given periodic function f(x) = 9 sin(6x + 5).
Solution:
Given periodic function is f(x) = 9 sin(6x+ 5)
Coefficient of x = B = 6
Period = 2π/ |B|, here period of the periodic function = 2π/ 6 = π/3
Example 2:
What is the period of the following periodic function?
f(a) = 6 cos 5a
Solution:
The given periodic function is f(a) = 6 cos 5a. We have the formula for the period of the function.
Period = 2π/B,
From the given, B = 5
Hence, the period of the given periodic function = 2π/5
Example 3:
Sketch the Graph of y = 4 sin(a/2).
Solution:
Given,
y = 4 sin(a/2)
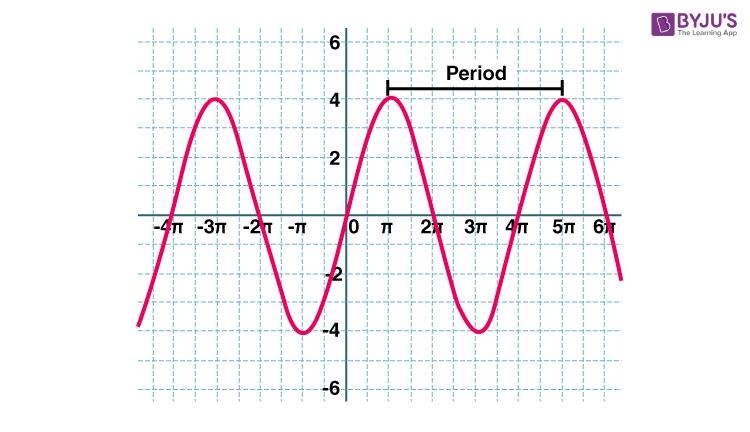
From the above graph, we can write the following:
- Period = 4π
- Axis: y = 0 [x-axis ]
- Amplitude: 4
- Maximum value = 4
- Minimum value = -4
- Domain: { x : x ∈ R }
- Range = [ -4, 4]
Practice Problems
- Find the period of the function f(x) = 3 cos 2x.
- Find the period and amplitude of the function y = 2 sin(6x – π) + 4.
- What is the period of the function y = 5 sin x – 7 sin 8x?
Keep visiting BYJU’S – The Learning App and download the app from the Google Play store and explore more videos to learn with ease.