Trigonometric Ratios Of Standard Angles
Trigonometric ratios are Sine, Cosine, Tangent, Cotangent, Secant and Cosecant. The standard angles for these trigonometric ratios are 0°, 30°, 45°, 60° and 90°. These angles can also be represented in the form of radians such as 0, π/6, π/4, π/3, and π/2. These angles are most commonly and frequently used in trigonometry. Learning the values of these trigonometry angles is very necessary to solve various problems.
Trigonometric Ratios Formulas:
The six trigonometric ratios are basically expressed in terms of the right-angled triangle.
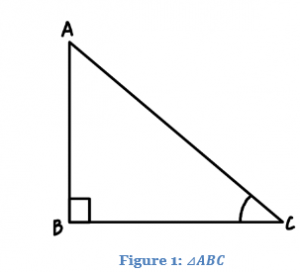
∆ABC is a right-angled triangle, right-angled at B (shown in figure 1). The six trigonometric ratios for ∠C are defined as:
\(sin~∠C\) = \(\frac{AB}{AC}\)
\(cosec~∠C\) = \(\frac{1}{sin~∠C}\)
\(cos~∠C\) = \(\frac{BC}{AC}\)
\(sec~∠C\) = \(\frac{1}{cos~∠C}\)
\(tan~∠C\) = \(\frac{sin~∠C}{cos~∠C}\)\(~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~\)
\(cot~∠C\) = \(\frac{1}{tan~∠C}\)
The standard angles for which trigonometric ratios can be easily determined are \(0°,30°,45°,60°\) and \(90°\). The values are determined using properties of triangles. The two acute angles of a right-angled triangle are complementary.
Trigonometric Ratios Table (Standard Angles)
| Angle = ∠C | 0° | 30° | 45° | 60° | 90° |
| \(sin~C\) | 0 | \(\frac{1}{2}\) | \(\frac{1}{\sqrt{2}}\) | \(\frac{\sqrt{3}}{2}\) | 1 |
| \(cos~C\) | 1 | \(\frac{\sqrt{3}}{2}\) | \(\frac{1}{\sqrt{2}}\) | \(\frac{1}{2}\) | 0 |
| \(tan~C\) | 0 | \(\frac{1}{\sqrt{3}}\) | 1 | \(\sqrt{3}\) | Not Defined |
| \(cosec~C\) | Not Defined | 2 | \(\sqrt{2}\) | \(\frac{2}{\sqrt{3}}\) | 1 |
| \(sec~C\) | 1 | \(\frac{2}{\sqrt{3}}\) | \(\sqrt{2}\) | 2 | Not Defined |
| \(cot~C\) | Not Defined | \(\sqrt{3}\) | 1 | \(\frac{1}{\sqrt{3}}\) | 0 |
The above table shows the important angles for all the six trigonometric ratios. Let us learn here how to derive these values.
Derivation of Trigonometric Ratios for Standard Angles
Value of Trigonometric Ratios for Angle equal to 45 degrees
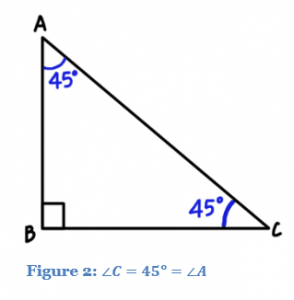
In \(⊿ABC\), if \(∠C\) = \(45°\), then \(∠A\) = \(45°\). Since the angles are equal, \(⊿ABC\) becomes a right angled isosceles triangle. So, \(AB\) = \(BC\). Assume \(AB\) = \(BC\) = \(a\) units.
Using Pythagoras theorem ,
\(AC^2\) = \(AB^2~+~BC^2\)
\(AC^2\) = \(a^2~+~a^2\)
\(AC\) = \(a\sqrt{2} ~units\)
\(∠C\) = \(45°\)
\(∴~ sin~∠C\) = \(sin~45°\) = \(\frac{AB}{AC}\) = \(\frac{a}{a\sqrt{2}}\) = \(\frac{1}{\sqrt{2}}\) \(~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~\)
\(cosec~45°\) = \(\frac{1}{sin~45°}\) = \(\sqrt{2}\)
\(cos~∠C\) = \(cos~45°\) = \(\frac{BC}{AC}\) = \(\frac{a}{a\sqrt{2}}\) = \(\frac{1}{\sqrt{2}}\) \(~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~\)
\(sec~45°\) = \(\frac{1}{cos~45°}\) = \(\sqrt{2}\)
\(tan~45°\) = \(\frac{sin~45°}{cos~45°}\) = \(\frac{\frac{a}{\sqrt{2}}}{\frac{a}{\sqrt{2}}}\) = \(1\)\(~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~\)
\(cot~45°\) = \(\frac{1}{tan~45°}\) = \(1\)
Value of Trigonometric Ratios for Angle equal to 30 and 60 degrees
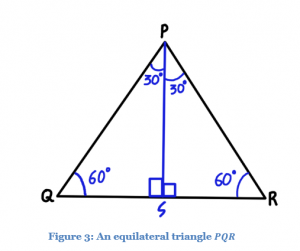
In figure 3, \(∆PQR\) is equilateral. The perpendicular from any vertex on the opposite side is coincident with the angle bisector of that particular vertex. Also, the perpendicular bisects the opposite side. If a perpendicular \(PS\) is dropped on \(QR\), then \(∠QPS\) = \(∠SPR\) = \(30°\) and \(QS\) = \(SR\). Assume \(PQ\) = \(QR\) = \(RP\) = \(2a\) units.
\(⇒~QS\) = \(SR\) = \(a\) units
In \(∆PSQ\), by Pythagoras theorem,
\(PQ^2\) = \(QS^2~+~PS^2\)
\(PS^2\) = \((2a)^2~-~a^2\)
\(PS\) = \(\sqrt{3a^2}\) = \(\sqrt{3} a\)
\(∠SPQ\) = \(30°\)
\(sin~∠SPQ\) = \(sin~30°\) = \(\frac{SQ}{PQ}\) = \(\frac{a}{2a}\) = \(\frac{1}{2}\) \(~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~\)
\(cosec~30°\) = \(\frac{1}{sin~30°}\) = \(2\)
\(cos~∠SPQ\) = \(cos~30°\) = \(\frac{PS}{PQ}\) = \(\frac{\sqrt{3} a}{2a}\) = \(\frac{\sqrt{3}}{2}\) \(~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~\)
\(sec~30°\) = \(\frac{1}{cos~30°}\) = \(\frac{2}{\sqrt{3}}\)
\(tan~30°\) = \(\frac{sin~30°}{cos~30°}\) = \(\frac{\frac{1}{2}}{\frac{\sqrt{3}}{2}}\) = \(\frac{1}{\sqrt{3}}\) \(~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~\)
\(cot~30°\) = \(\frac{BC}{AB}\) = \(\sqrt{3}\)
Similarly, ratios of 60° are determined by finding the ratios of \(∠SQP\) as
\(sin~60°\) = \(\frac{\sqrt{3}}{2}\) \(~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~\)
\(cos~60°\) = \(\frac{1}{2}\)
\(tan~60°\) = \(\sqrt{3}\) \(~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~\)
\(cot~60°\) = \(\frac{1}{\sqrt{3}}\)
\(cosec~60°\) = \(\frac{2}{\sqrt{3}}\) \(~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~\)
\(sec~60°\) = \(2\)
Value of Trigonometric Ratios for Angle equal to 0 and 90 degrees
In \(∆ABC\) is a right angled triangle. If the length of side \(BC\) is continuously decreased, then value of \(∠A\) will keep on decreasing. Similarly, value of \(∠C\) is increasing as length of \(BC\) is decreasing. When BC = 0, ∠A = 0 , ∠C = 90° and AB = AC.
Taking ratios for \(∠A\) = \(0°\)
\(sin~∠A\) = \(sin~0°\) = \(\frac{BC}{AC}\) = \(\frac{0}{AC}\) = \(0\)
\(cosec~0°\) = \(\frac{1}{sin~0°}\) = \(\frac{1}{0}\) = Not Defined
\(cos~∠A\) = \(cos~0°\) = \(\frac{AB}{AC}\) = \(\frac{AC}{AC}\) = \(1\)
\(sec~0°\) = \(\frac{1}{cos~0°}\) = \(\frac{1}{1}\) = \(1\)
\(tan~0°\) = \(\frac{sin~0°}{cos~0°}\) = \(\frac{0}{1}\) = \(0\)
\(cot~0°\) = \(\frac{1}{tan~0°}\) = \(\frac{1}{0}\) = Not Defined
Taking ratios for \(∠C\) = \(90°\)
\(sin~∠C\) = \(sin~90°\) = \(\frac{AB}{AC}\) = \(\frac{AC}{AC}\) = \(1\)
\(cosec 90°\) = \(\frac{1}{sin~90°}\) = \(\frac{1}{1}\) = \(1\)
\(cos~∠C\) = \(cos~90°\) = \(\frac{BC}{AC}\) = \(\frac{0}{AC}\) = \(0\)
\(sec~90°\) = \(\frac{1}{cos~90°}\) = \(\frac{1}{0}\) = Not Defined
\(tan~90°\) = \(\frac{sin~90°}{cos~90°}\) = \(\frac{1}{0}\) = Not Defined
\(cot~90°\) = \(\frac{1}{tan~90°}\) = \(\frac{0}{1}\) = \(0\)
Following is the trigonometric ratios table which contains all the trigonometric ratios of standard angles:
Solved Examples
Question 1: What is the value of tan 30+sin 60?
Solution: tan 30 = 1/√3 and sin 60 = √3/2
Adding both the values we get;
1/√3 + √3/2
Rationalising the denominator gives:
(2+√3.√3)/2√3
2+3/2√3
5/2√3
Question 2: What is the value of sin45 – cos 45?
Solution: Sin 45 = 1/√2 and cos 45 = 1/√2
Therefore, on putting the values we get:
1/√2 – 1/√2 = 0
Video Lesson
Trigonometric Ratios of Compound Angles

To learn about other topics, visit www.byjus.com. Download BYJU’S – The Learning App to enjoy interactive learning.