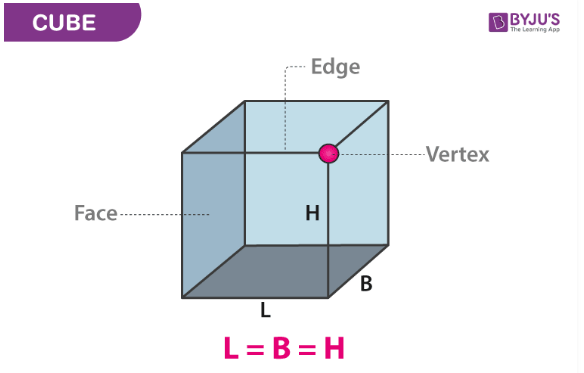
Volume of a cube is the total cubic units occupied by it, in a three-dimensional space. A cube is a 3d-shape, that has six faces, twelve edges and eight vertices. Hence, the volume of a cube is the space enclosed by its six faces. Unlike, 2d shapes, it has additional dimensions apart from length and width, which is called height or thickness.  Therefore, the volume of cube is equal to product of its length, width and height. It is measured in cubic units. The more the value of its dimensions the more is the volume of cube.

In this article, we will discuss the definition and formula of volume of a cube along with examples.

What is the Volume of a Cube?

Definition: The volume of a cube defines the number of cubic units, occupied by the cube completely. The unit of volume of the cube is in cubic units such as centimeters³, meters³, inches³, feet³, etc.

A cube is a solid three-dimensional figure, which has 6 square faces or sides. The volume of a cube will be the total space occupied by it. Since all the faces of the cube are square in the shape, hence, the length of edges will also be equal. Therefore, the length, width and height of the cube are of equal length.

If length, width and height of cube is equal to ‘a’, then;

Volume of cube = a × a × a

If we know the edge length i.e. “a”, then we can find the volume of the cube. Let us learn how to find the volume of any cubical structure.

Volume of a Cube Formula

We can easily find the volume of the cube (V), by knowing the length of its edges. Suppose, the length of the edges of the cube is ‘a’. Then the V will be the product of length, height, and width. So, the volume of the cube formula is:

Volume of a Cube = Length × Width × Height

Volume = a × a × a

Volume = a³

Where ‘a’ is the length of the side of the cube or edges.

Derivation for Volume of a Cube

The volume of an object is defined as the amount of space a solid occupies. We know that a cube is a 3-dimensional object whose all the sides i.e. length, breadth, and height are equal. Now for a cube, the volume derivation will be as follows:

• Consider a square sheet of paper.
• Now, the area that the square sheet will take up will be its surface area i.e. its length multiplied by its breadth.
• As the square will have equal length and breadth, the surface area will be “a²“.
• Now, a cube is made by stacking multiple square sheets on top of each other so that the height becomes “a” units. This gives the height or thickness of the cube as “a”.
• Now, it can be concluded that the overall area covered by the cube will be the area of the base multiplied by the height.
• So, Volume of Cube = a² × a = a³

How to Find Volume of a Cube?

There are two methods by which we can find the volume of a cube.

• Using Edge-length
• Using Diagonal

Both the methods are formula-based and easy to understand.

Volume of Cube Given the Edge-Length

By the formula of volume of a cube, we know that;

Volume = (Edge of the cube)³

Therefore, if we know the edge length of the cube, we can easily find its volume.

Example: If edge-length is 3 cm, the volume of the cube;

Volume = 3³ = 3 x 3 x 3 = 27 cu.cm.

Volume of a Cube Given the Diagonal

The volume of a cube whose diagonal (d), is given is given by:

Hence, by measuring the length of the diagonal of cube, we can evaluate the volume of cube.

Example: If length of diagonal of cube is 3 inches, then the volume will be:

Volume = √3 × d³/9 = √3 × 3³/9 = 3√3 cu.in.

Check: Diagonal of a Cube Formula

Video Lesson on Volume of Cube

For More Information On Volumes of Cubes and Cuboid, Watch The Below Video:

Surface Area of a Cube

The surface area of the cube is the total area occupied by its outer surface. We can find the surface area of a cube, which is equal to the number of square units that cover the surface of the cube, completely. The general formula of surface area for a cube of side ‘a’, is given by;

Surface Area of Cube = 6a²

Related Articles

• Cube
• Volume
• Cube and Cuboid
• Surface Area Of Cube
• Volume of a Cone
• Volume Of Cuboid
• Volume of a Cylinder
• Volume Of Sphere
• Volume of a Pyramid
• Volume of a Prism

Solved Examples on Volume of Cube

Question 1: Find the volume of the cube, having the sides of length 7 cm.

Solution:

Given, the length of the sides of the cube is 7 cm.

We know, Volume of a cube = (length of sides of the cube)³

Therefore, Volume, V = (7 cm)³

V = 343 cm³

Question 2: Find the length of the edges of the cube, if its volume is equal to 125 cm³.

Solution:

Given, Volume of the cube = 125 cm³.

Let the length of the edges is ‘a’.

We know, by the formula,

The volume of a cube = (length of edges of the cube)³

Substituting the value, we get,

125 = a³

Or a = ³√125

Or a = 5 cm

Therefore, the length of the cube is 5 cm.

Question.3: What is the volume of cube, whose length of diagonal is 27 cm.

Solution: By the formula of volume of cube given the diagonal, we have;

Volume = √3 × d³/9

d = 27 cm (given)

Therefore,

Volume of cube = √3 × 27³/9

= √3 × (27 × 27 × 27)/9

= √3 × (27 × 27 × 3)

= 3788 (Approx)

Practice Questions

1. Find the volume of cube with edge-length equal to:
   • 4 cm
   • 7 in
   • 11 m
   • 10 ft
2. What is the volume of cube if length of diagonal is 15 cm?

Learn about different geometrical shapes and sizes here in BYJU’S and also download its app to get personalized and interesting videos.